
The patterns studied here are formed by combining 16 square tiles all decorated with the same pattern
of two quarter-circles drawn in opposite corners. This was sold at one time as a mini-puzzle by BHS 1993,
with four colours of markings, made by Lagoon Games, P.O.Box 311, Kingston KT2 5QW.

Each piece has two orienations.
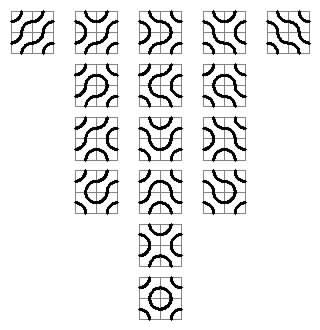
So the total of oriented 2×2 patterns is 2^4 = 16 as shown below.
The one on the right reverses all four pieces. The four in the second column reverse one of the pieces,
and those in the fourth column reverse three pieces, while the six in the middle each reverse two pieces.
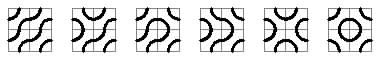
There are just six geometrically distinct 2×2 patterns (i.e. disregarding rotations and reflections).
The total of oriented 4×4 patterns is 2^16 = 65536.
Among these the number with octonary symmetry is 8 (2^3 = 8) as shown below. Each has only one orientation.
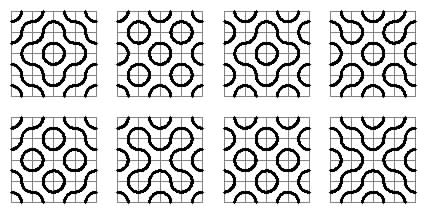
Geometrically distinct patterns with two axes of symmetry, but not 4,
consist of 2^2 = 4 with lateral axes (in the left column here)
and 2^4 = 16 with diagonal axes. Each of these can appear in two different orientations.
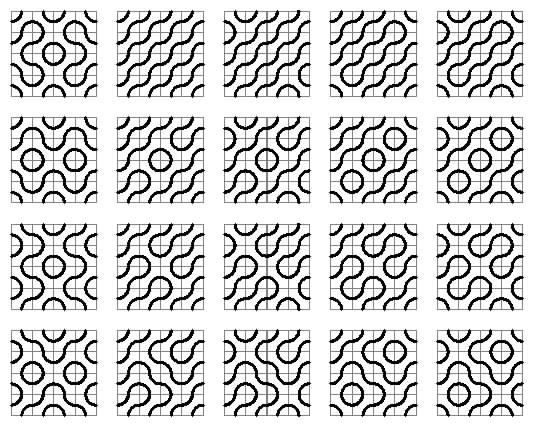
There are also 4 patterns that show 90 degree rotary symmetry without axes.

Number with lateral axis is 2^8 = 256 oriented. This includes the 8 with octonary symmetry plus 124 pairs.
Number with diagonal axis is 2^10 = 1024 oriented. This includes the 8 with octonary symmetry plus 508 pairs.
Number with 180 degree rotary symmetry is 2^8 = 256 oriented. This includes the 8 with octonary symmetry
and the 4 with 90 degree rotary symmetry plus 120 pairs.
Examples with binary symmetry:
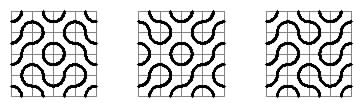
So the total symmetric is 8 + 4 + 124 + 508 + 120 = 764.
And the total asymmetric is (65536 - 8 - 2×4 - 3×124 - 4×508 - 4×20)/8 = 7814.
Total geometrically distinct is 764 + 7814 = 9578.
These numbers have not been independently checked.
Readers may like to extend these patterns to larger squares.