The Brocard Centres of a Triangle
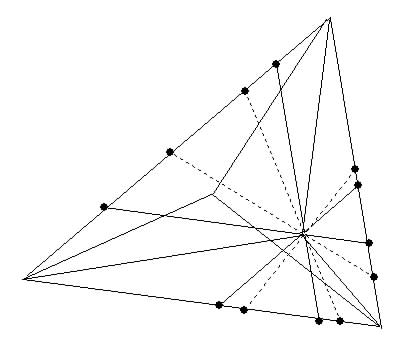
There are six circles that pass through two vertices of a triangle and are tangent to one of its sides. The tangency must occur at one of the vertices passed through. These six circles have two triple points of intersection, known as the Brocard Points of the triangle. They are always inside the triangle and coincide only when the triangle is regular, in which case the six circles reduce to three.
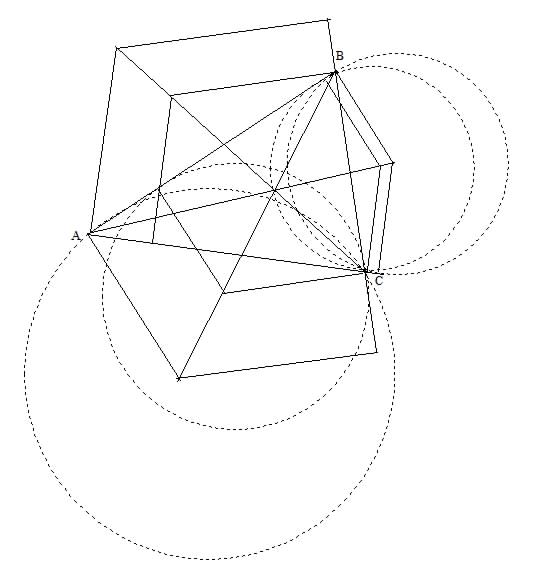
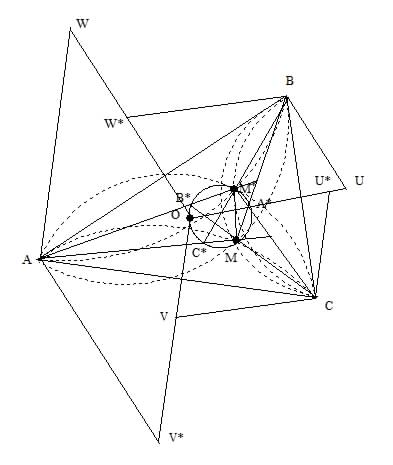
M is the intersection of the circles centred at U, V, W (where the perpendiculars from A, B, C are drawn clockwise) and M* of those centred at U*, V*, W* (anticlockwise). These centres lie in pairs on the external parts of the side-bisectors. The join MM* is the Brocard Line of the triangle.
The six circles form three crescents with cusps at the vertices of the triangle. In the isosceles case the crescent at the base reduces to one arc, and in the regular case the six reduce to three.
There is a unique angle w such that lines drawn through A, B, C at the angle w to AB, BC, CA concur. It follows that the similar lines through A, B, C at angle w to AC, BA, CB also concur. The points of concurrence are the Brocard Points, and the Brocard angle w is given by cotw = cota + cotb + cotg.
We also have AM.BM.CM = AM*.BM*.CM*.
MM* is perpendicular to OO* where O is the circumcentre and O* the Lemoine centre. M and M* lie on the circle on OO* as diameter (the Brocard circle) which also passes through the points of intersection BM.CM*, CM.AM*, AM.BM*, which we denote as A*, B*, C*.
What is the angle subtended by MM* at O (and B*, C*) is it w?
The midpoint of MM* is the incentre.