Definition of Centre
A regular triangle has one undisputed centre, namely its
centre of symmetry, which has many properties that in a scalene triangle are
possessed by separate points. For example, the centre of symmetry is the point of intersection of the lines joining the vertices to the midpoints
of the opposite sides. These lines are also perpendicular to the sides and also bisect the angles at the vertices.
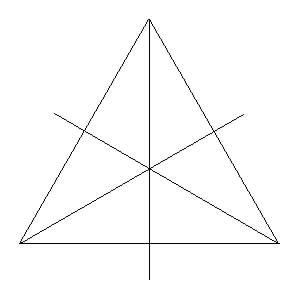
In a scalene triangle these three properties determine three distinct sets of lines and three distinct points of concurrency (the midcentre,
orthocentre and incentre). Thus by a
centre of a scalene triangle we mean any point found by a construction which, when applied to a regular
triangle produces the centre of symmetry.
Centres in General
The reflection of a line through a vertex in the bisector of the angle at that vertex is called its isogonal line.
Thus AX and AY are isogonals if BAX = CAY or BAY = CAX.
The isogonals of three concurrent lines are themselves concurrent. These pairs of points are called isogonal conjugates of each other.
A Survey of the Centres of a Triangle
1. The medians meet at the midcentre or centroid.
The centroid is the centre of mass of a uniform triangular region.
2. The bisectors of the angles meet at the incentre.
Related points are the Gergonne centre and Nagel centre and the three "excentres".
3. The Morley centre is the centre of the regular triangle of points formed by pairs of angle trisectors.
4. The Symmedian or Lemoine Centre is the point where the isogonals of the medians (the symmedians) meet, G'.
(the isogonal conjugate of the centroid G).
5. The Fermat Centre is where lines from the vertices form a star (that is the angles between them are all 60°).
6.
