Triangles

General Properties

The sum of the lengths of any two sides of a triangle is greater than the length of the third side. (Otherwise they would form a shorter path than the straight one formed by the third side).

Two triangles are congruent if they have the following features the same: (a) three sides, (b) two sides and included angle, (c) one side and two angles in the same relative position.

The greater side of a triangle has the greater angle opposite it, and vice versa. Two sides of a triangle are equal if and only if the two angles opposite the sides are equal, and vice versa.

An asymmetric triangle is called scalene, its sides are all of different lengths and its angles are all of different magnitudes. A singly symmetric triangle is isosceles, it has two sides equal and two angles equal. A doubly symmetric triangle is a regular polygon, it has all sides equal (i.e. it is equilateral) and it has all angles equal (i.e. it is equiangular).

The angles in a triangle add to half a cycle (i.e. 180°). An external angle of a triangle is equal to the sum of the other two internal angles (the internal and opposite angles).

In a general triangle A, B, C with sides a, b, c and opposite angles a, b, g if h is the perpendicular from C to c we have, by defnition of the sine function, sina = h/b and sinb = h/a from which it follows that a/b = sina/sinb. And by symmetry similar relations hold between the other pairs of angles and sides. In other words the lengths of the sides are proportional to the sines of the angles they subtend.

In the general triangle we also have c² = a² + b² + 2.a.b.cosg, which is a generalisation of the theorem of Pythagoras. When g is a right angle the cosine is zero.

Areal Coordinates

If {A, B, C} is a triangle of points and P is any other point in the same plane, the position of P relative to A, B, C can be described by numbers (p, q, r) expressing the areas of the triangular regions PBC, PCA, PAB as fractions of area ABC. These numbers are the areal coordinates of P with respect to A, B, C.

A = (1,0,0), B = (0,1,0), C = (0,0,1). On BC p = 0, on CA q = 0, on AB r = 0. Points inside the triangle have all coordinates positive. Points in the three-sided regions outside the triangle have one negative coordinate. Points in the two-sided outer regions have two coordinates negative. At least one coordinate must be positive.

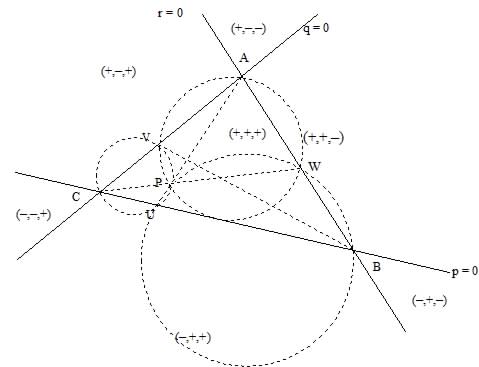
We always have p + q + r = 1. Given any two coordinates the third is determined; they are not independent. There are many triplets of numbers (p, q, r) that do not represent points because they fail to sum to unity, e.g. there is no point (0,0,0) and no point with three negative coordinates.

Three Points on a Triangle

If UVW are points on the sides of triangle ABC then the circles through AVW, BWU, CUV are concurrent, at a point P, called the pivot. [Pedoe p.430]

If UVW divide the sides in the ratios u,v,w then the lines AU, BV, CW form a triangle whose area is D(uvw – 1)²/(uv+u+1)(vw+v+1)(wv+w+1). [Routh's theorem].

AU, BV, CW concur if and only if uvw = 1 [Ceva's theorem]