Vandermondian Tours

«Quaternary Pseudotours

The first to construct a tour on the standard chessboard by joining up circuits arranged in quaternary symmetry was A. T. Vandermonde (1771), so it seems appropriate to call all such tours Vandermondian. Here we study ways of arranging circuits to form pseudotours with quaternary symmetry and then give examples of tours formed from them by simple-linking. There are many more cases that might be considered, but we focus on particular cases that are of historical interest or have special geometrical properties.

Vandermonde's paper of (1771) began with an arrangement of four asymmetric 16-move circuits arranged in direct quaternary symmetry [see (a) below], then as a first step he joined them in pairs to form two centro-symmetric 32-move circuits (each composed of two 16-move open paths). In terms of our ‘centrifugal’ cell numbering the 16-move corner-to-corner paths, both closed and open, are represented by the same sequence 94783102656138749. The path is closed or open according to which '2' the knight moves to following the '0'. There is also an apparent choice of '6' following the '2', but the wrong choice here leads the knight into the final corner from the wrong direction.

A peculiarity of the circuits chosen by Vandermonde is that by reflecting them in a diagonal they can also be arranged in oblique quaternary symmetry [see (c) below], as noted by H. E. Dudeney (Amusements in Mathematics 1917). For it to be possible to arrange four identical 16-move paths in both direct and oblique quaternary formation, the pattern of cells occupied by two diametrally paired paths must be reflectible in a diagonal, as well as being superposable by a half-turn. These two properties combine to ensure that it must also be reflectible in the other diagonal, i.e. the pattern of cells (but not the pattern of moves) has both diagonals as axes of symmetry. There are ten other formulae that give four pseudotours related in the same way as in Vandermonde's example.

All possible ways of covering the 8×8 board with two or four congruent circuits to form quaternary symmetric pseudotours were listed by the Abbé Philippe Jolivald under the alias of Paul de Hijo (1882). For the cases of oblique symmetry he found 140 arrangements of four 16-move circuits and 150 arrangements of two 32-move circuits. For the cases of direct quaternary symmetry he found 368 arrangements of four 16-move circuits and 378 arrangements of two 32-move circuits (although the 368 total was miscounted by him as 301 and this error was copied by E.Lucas and other subsequent writers). T. W. Marlow confirmed the figures in a computer study reported in Chessics (1985 p.92).

The following tables of the numbers of paths between particular squares are adapted from Marlow's results. It is assumed that the path starts in a corner (9)-(4). The next move is then to any of the cells numbered (0), (1), (3), (6), (7) and the path continues until the 15th cell reached is also one of the cells numbered (0), (1), (3), (6), (7) that is a knight's move from the appropriate (4) that connects either to the initial corner for the closed 16-move circuits, or the opposite corner for the open paths which combine to form the 32-move circuits. An ‘appropriate’ (4) is one that is related to the initial (4) by reflection in a diagonal.

Chart of numbers of quaternary pseudotours

(a) Direct Closed. - (0) (1) (3) (6) (7) (0) 0 18 16 65 36 (1) - 2 7 20 24 (3) - - 2 41 12 (6) - - - 43 63 (7) - - - - 19 total 368	(b) Direct Open. - (0) (1) (3) (6) (7) (0) 0 16 24 70 32 (1) - 2 4 21 23 (3) - - 4 38 12 (6) - - - 43 70 (7) - - - - 19 total 378
(c) Oblique Closed. - (0) (1) (3) (6) (7) (0) 0 3 7 12 13 (1) - 0 3 15 6 (3) - - 3 15 8 (6) - - - 16 25 (7) - - - - 14 total 140	(d) Oblique Open - (0) (1) (3) (6) (7) (0) 0 3 13 15 17 (1) - 1 2 12 9 (3) - - 6 13 4 (6) - - - 13 27 (7) - - - - 15 total 150

It is proposed to include a complete illustrated catalogue of all these quatrenary pseudotours in due course.

«Simple-Linking of Vandermonde's Circuits

To find all the ways of simple-linking the Vandermonde circuits we proceed as described in the page on simple-linking of pseudotours, giving each circuit a label, a, b, c, d, the same letter on all cells visited by the same circuit; then the problem is to find four moves aa, bb, cc, dd that can be linked. I have found seven geometrically distinct ways of simple-linking Vandermonde's original array of four circuits. Four of these are symmetric and are shown below. Two of these, in which the linkage polygon is a hexagon, with two sides of two-move length, were given by Jaenisch (1862, Figs 86 p.13 and 87 p.16). It should be noted that though these two linkage polygons are geometrically congruent they are related by reflection in a diagonal and therefore produce different tours, since the array of four circuits is altered by diagonal reflection. The linkage octagons are indicated by a grey background line. The other three asymmetric cases are: (c8e7f5e3g4e5c6a7), (c7d5e3g2e1d3b4a6), (c7e6d4e2f4d3b4a6).

Applying the same methods as above to the Vandermonde circuits in their rotary formation we also find seven tours by simple linking, four again symmetric, and there are again two hexagon-shaped linkage polygons which give distinct tours. We give diagrams of these four tours. The other three asymmetric linkage polygons are: (a7c8e7f5e3g4e5c6), (a6c7e6d4e2f4d3b4), (a6c7d5f4g2e1d3b4).

«Quarterboard Tours: Aladdin's Conundrum

As mentioned in the history pages, the leading chess player at the court of the emperor Timur (1336–1405), Ala'addin Tabrizi, wrote a treatise on chess that may survive in part in the form of a manuscript in the library of the Royal Asiatic Society, London, and includes the question of making a closed tour on a quarter of the chessboard, but without a solution. A tour of a 4×4 quadrant is impossible, but a tour of a quarter board of special shape is possible. Surprisingly this quarterboard is the ONLY one that has a closed tour, and moreover it can be toured ONLY in the one way! The result is unique.

Whether the author of the manuscript knew of this result we may never know for sure, but it makes a good story, appropriate to the name of Aladdin. The earliest source in which this quarter tour is definitely to be found is de Hijo (1882), who gives the tour in his catalogue of all the possible 16-move knight circuits in oblique quaternary symmetry, and makes special note of it, though he does not give a diagram of it. It was rediscovered independently by H. E. Dudeney (1917) who made it the basis of several puzzles.

By deleting one move from each quarter tour and re-linking we can form five different closed tours of the whole board. The three symmetric forms are shown below. One of these was discovered independently by C. T. Blanshard and published in Chess Amateur August 1923. In the two asymmetric forms the linkages are (b7a4b3c1d3e5f7d8b7) and (b7a4b3c1d3e5f7d6b7).

H. E. Dudeney also found another 16-move circuit that covers a quarter of the board and can be repeated four times in oblique quaternary symmetry without intersecting its duplicates. The area it covers however does not make a connected board, since one of the squares is attached only at a corner. This pseudotour generates 9 tours by simple linking, 3 of which are symmetric. The asymmetric linkages are (a5-c6/b7, d8-f7, g5-f3, e5-c4), (c6-e5, f3-d2, e4-g5, e6-d4), (b5-c3, e4-g5, f7-e5, f3-d4), (a5-c6, d4-f5, h6-g4, e5-c4) (and one other?)

The last example here led me to ask: Is it possible to find a set of four circuits in quaternary symmetry in which one of the linkage polygons is the complete ‘circle’ of knight moves. The answer is No, but it is possible on the 12×12 board though the resulting tour is not symmetric.

«Non-Intersecting Circuits in Quaternary Symmetry

There are just three of the 16-move circuits in oblique quaternary symmetry that do not intersect themselves. The following diagrams show symmetric tours derived from these by simple linking.

A.

B.

C. This case is remarkable as being linkable by a star polygon in two ways (to give asymmetric tours). Three symmetric linkings are also shown, one of which leaves the centre unaltered.

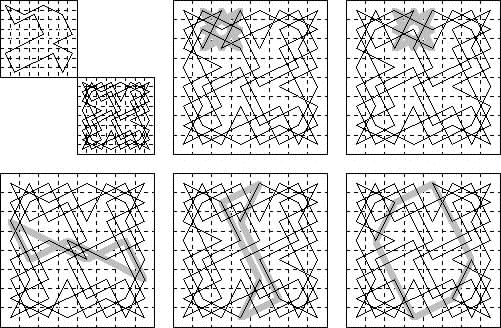
D. Only one other pseudotour is linkable by a star polygon, as in this last diagram. This pseudotour path intersects itself once.