
Under this heading we collect together tours which have geometrical properties that are shown by some but not all the moves, such as formation of circuits or angles — as opposed to geometrical properties depending on all the moves, such as symmetry. In some the required properties stand out clearly and do not need emphasising, others are helped by emphasising certain lines, or require an explanatory caption.
The chess knight moves in four different directions, or in eight different orientations, depending on whether we take account of the sense of movement along the lines.
An 8×8 tour with no moves in one of the four directions, say parallel to a8-c7, is impossible, since at the a8 and h1 corners only one move would be available; but these cells cannot be the end-points of a tour since they are of the same colour. An 8×8 tour with only one move in a given direction is possible. Kraitchik (1927) gave an example, which I have improved on by making the odd move the last (or first) move in the tour. In a closed 8×8 tour there must be at least two moves in each direction. I show a tour with just two moves in one direction; it is also symmetric.

The maximum moves in one direction appear to be 37 in an open tour, by E. Lange (Sphinx June 1931) and 34 in a closed tour, which may be symmetric. (The Lange example also shows the maximum number of non-intersected moves: 16.) If we require the moves we count not to be joined up into lines the maximum in a given direction reduces to 28, as shown in a tour (probably taken from some earlier source) used for a crypotour puzzle by T. B. and F. F. Rowlands (Chess Fruits 1884). The directional property was not pointed out there. It shows 54 single moves in two directions, 28 in one, 26 in the other. This was converted by H. J. R. Murray into a closed tour with 27 in each direction.
The first diagram below, by E. Lange, solves the problem of constructing a tour with 16 moves in each of the four directions. This is not quite symmetric, as the four darker moves indicate; in fact we can prove that a symmetric solution of this problem is impossible.
|
Theorem: A symmetric tour with 16 moves in each direction is impossible.
|
It follows that it is impossible to construct a symmetric tour with 8 moves of each of the eight oriented types: (±1, ±2), (±2, ±1). However I have recently shown that a solution of this long-standing problem (mentioned by Murray in his 1942 ms) is possible in an asymmetric tour. The example given here was constructed from Roget's four nets using the linkage polygon of alternate straights and slants shown, in which the deleted straights and the inserted slants (shown darker) occur in similarly oriented pairs.

See the Games and Puzzles Journal issue 23 (2002) for a more colourful rendering of this tour which shows the moves as arrowed lines.
The problem of showing straight lines of moves has been one of the most intensively studied, at least on the 8×8 board. Parmentier (1891) showed an example with nine three-move lines in an open tour, but the maximum of ten was first achieved by Dr Hogrefe in Weser Zeitung 13 July 1924. T. R. Dawson gave another example in Problemist Fairy Chess Supplement February 1932 and V. Onitiu reported examining all possible arrangements of ten lines on the 8×8 board, 1330 in all, and found that only six of them admit tours (PFCS June 1932).

These six cases admit slight variations, making a total of 12 geometrically distinct solutions. For example a variation of Hogrefe's solution, adding a 2-move line a1-c5, is: (a) delete h4-g2, b3-a5, c5-b7 (b) insert a5-b7, b3-c5, e1-g2. This increases the number of 'straight angles' occurring to 21. This total is also achieved in the fourth example. It is possible to achieve 10 three-unit lines parallel, or 10 three-unit plus 2 two-unit parallel, in incomplete tours of the 8×8 (T.R.Dawson PFCS 1932, problem 459), and V.Onitiu achieved 10 three-unit lines with 8 parallel in an incomplete tour omitting only one cell.
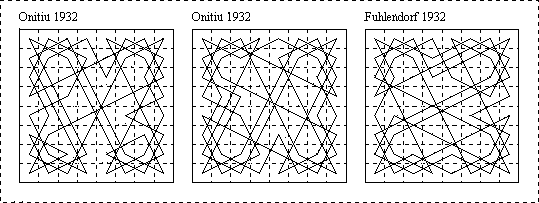
In a closed tour the number of three-unit lines possible reduces to nine. Three examples from PFCS are shown; two by V. Onitiu and one by G. Fuhlendorf.
In a symmetric tour the number reduces to eight. This was first shown by E. Bergholt, British Chess Magazine, March 1918. As mentioned there, but not diagrammed, G. L. Moore found two other solutions; I show the diagrams from the manuscript Moore sent to Murray in 1920. Other composers have subsequently found these three eight-line tours independently. Moore's first example also includes two two-move lines, making the number of "straight angles" 18. Moore (1920) also explored the number of symmetric tours with six three-unit lines and reported finding 173.

The maximum numbers of two-unit lines achieved are 15 in an open tour (E. Lange, Sphinx August 1931), 14 in a closed tour, 13 in parallel in an open tour (J. Akenhead Fairy Chess Review October 1946) and 12 in a symmetric tour.

I insert here also my tour showing all 21 geometrically distinct positions of the knight's move with respect to the board, constructed to present an enhanced form of the Knight's Tour as Conjuring Trick (Chessics, #22, p.66, 1985). By memorising this tour it is possible to allow the spectators to place a white and a black knight, attacking each other, anywhere on the board, and for the conjuror to then move either of the knights to capture the other, after first visiting all the other cells on the board.
Another problem of interest is the construction of tours with consecutive three-unit or two-unit lines. Murray (1942) gives examples showing four consecutive three-unit lines in a closed tour and three in a symmetric tour. The 4-line formation can be placed in a second position, as shown in my alternative solution.
