
This page was begun on 28 October 2015, revised 17 November 2015.
The only form of overall symmetry possible in a knight tour on the 8×8 chessboard is that of rotation by 180 degrees. Other symmetries can be approximated to but there are always a few moves that do not match. The following collection now consists entirely of tours of my own composition, to show all possible central angle patterns. Of these tours 55 were already in my files, some dating back to the 1970s, 82 were newly composed Aug-Oct 2015, the remaining 46 in Nov 2015. Let me know if you spot any in earlier sources. An absorbing recreation is to create your own collection of such tours! See note at end on historical examples.
The following diagrams explain the lettering method for the central angles which I developed originally in Chessics 1985 (#22 p.70) for classifying tours on the 6 × 6 board.

No closed tours are possible on the 8×8 board using the angle (a) because of the formation of a 4-move circuit with the moves through the adjacent corner cell, and no symmetric tours are possible on any board using the angle (m) since two diametrally opposite (m)s form a 4-move circuit. This reduces the number of different angles available to 14.
In the case of a symmetric tour the angles in diametrally opposite cells will be the same. Thus in the centre four cells there can be at most only two different angles. We can thus denote the central pattern by the names of these two angles, taken in alphabetical order. There are 14 cases with the four angles the same, ranging from (bb) to (pp), and (14×13)/2 = 91 with two different angles, ranging from (bc) to (op). Making 105 cases in all.
The angles (a), (h), (m), (p) are symmetric by reflection in the diagonal that bisects them. In the case of the asymmetric angles I call the move nearest the corner (i.e. forming part of an a, h or m angle) the leading arm of the angle, shown in bold in the above diagrams. This enables us to distinguish two formations made with the same asymmetric angle as: direct (=) or oblique (~), according as the leading arms of the angles are similarly oriented (both vertical or both horizontal) or differently oriented (one vertical the other horizontal). Thus the angle pattern (bb) occurs in two forms (bb=) and (bb~). Roughly speaking the angles are related by reflection or rotation. It will be found further that this distinction applies in all cases, even when the two angles are different.
Thus we would now appear to have 210 cases in all. However tours involving the symmetric angles h or p occur in only one form, there is for example only one pattern (bh) with no distinction between (bh=) and (bh~), since h and p always have one arm vertical and the other horizontal. This effect reduces the total by 27, namely three cases using the angles h and p only: (hh), (hp), (pp). Then there are 12 cases using h with an asymmetric angle, and similarly 12 cases using p. This makes the number of cases 210 – 27 = 183.
I show one tour for each case and list them in alphabetical order for ease of reference. Each tour now has a separate diagram, so that they can easily be replaced if I find an improved version. There are many more examples of each case of course, because the moves between the centre and the corners can be varied considerably. Some centres allow more variation than others.
bb=, bb~, bc=, bc~




bd=, bd~, be=, be~



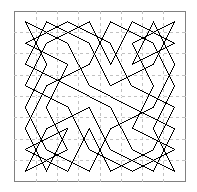
bf=, bf~, bg=, bg~, bh





bi=, bi~, bj=, bj~



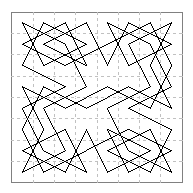
bk=, bk~, bl=, bl~



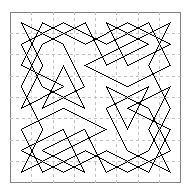
bn=, bn~, bo=, bo~, bp





cc=, cc~, cd=, cd~




ce=, ce~, cf=, cf~


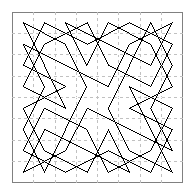

cg=, cg~, ch

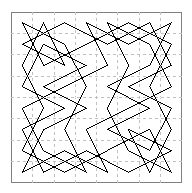

ci=, ci~, cj=, cj~




ck=, ck~, cl=, cl~




cn=, cn~, co=, co~, cp



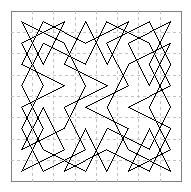

dd=, dd~, de=, de~




df=, df~, dg=, dg~, dh




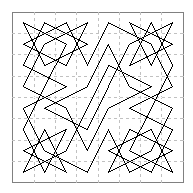
di=, di~, dj=, dj~
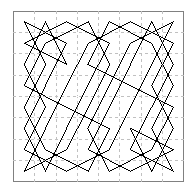


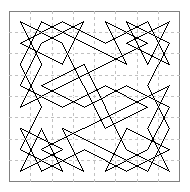
dk=, dk~, dl=, dl~
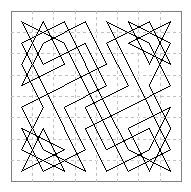

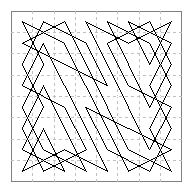

dn=, dn~, do=, do~, dp

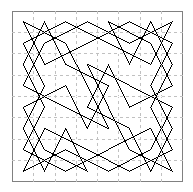

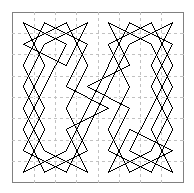

ee=, ee~, ef=, ef~




eg=, eg~, eh

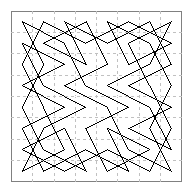

ei=, ei~, ej=, ej~




ek=, ek~, el=, el~




en=, en~, eo=, eo~, ep



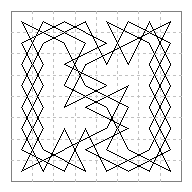

ff=, ff~, fg=, fg~, fh





fi=, fi~, fj=, fj~




fk=, fk~, fl=, fl~



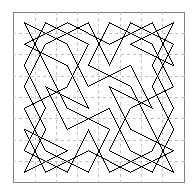
fn=, fn~, fo=, fo~, fp





gg=, gg~, gh



gi=, gi~, gj=, gj~
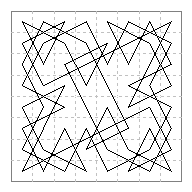
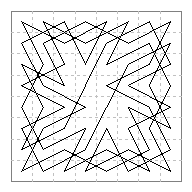


gk=, gk~, gl=, gl~




gn=, gn~, go=, go~, gp
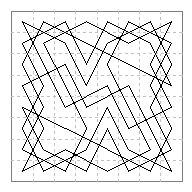




hh, hi, hj, hk



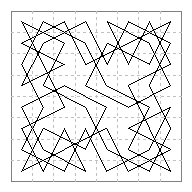
hl, hn, ho, hp



ii=, ii~, ij=, ij~




ik=, ik~, il=, il~



in=, in~, io=, io~, ip





jj=, jj~, jk=, jk~

jl=, jl~, jn=, jn~




jo=, jo~, jp



kk=, kk~, kl=, kl~




kn=, kn~, ko=, ko~, kp




ll=, ll~, ln=, ln~




lo=, lo~, lp



nn=, nn~, no=, no~, np




oo=, oo~, op, pp




Some notes on the structure of particular tours.
(bg~) (dl~) (kl=) Rectangular half-board tours, i.e. joining two 4 by 8 tours.
(bb~) (bh) (bl~) (df~) (dk=) (dl=) (do~) (il=) (jl~) Shaped halfboard tours,
i.e. there is a line from centre to edge cutting the tour once only.
(bg=) (io=) Maximum 48 moves in octonary symmetry.
(be=) (bg~) Squares and diamonds tours.
(fi~) (no=~) Double linking from collini circuits.
(bh) (ce=) (de=) (dl~) (kl=) Simple linking from crosspatch patterns.
(dg~) (gi~) (gk~) (kk~) Simple linking from quaternary noncrossing circuits.
(bd=) (fg=~) (gj=) (hh) (hl) Simple linking from octonary pseudotours.
Also (hh) includes three successive two-move lines twice.
(bf=) (bj=) (fh) Double cross tours.
(in~) (jn~) Centre-circling tours.
(cf~) (ii=) (jj~) (jk=) (kk=) (nn=) Show mixed quaternary symmetry.
The following are the 46 tours from historical sources that were previously included, in chronological sequence: Nilakant-ha 1640 (bj~). Euler 1757 (eg=). Euler 1759 (bc=) (cc=) (cd=) (cg=) (el~) (eh) . Kafer 1842 (dk=) (jj~). Brede 1844 (bb~) (cc~) (dd=) (ee~) (ff~) (gg=~) (go=) (ll~) (lp) (nn~) (oo~). Tomlinson 1845 (fi=). Wenzelides 1849 (cn~) (co=) (dd~) (di=) (dp) (fl=) (gh) (hj) (ii~) (il~) (in=) (jp) (kn=) (ln=) (pp). Jaenisch 1862 (kn~). Adam 1867 (be~) (bo=) (de~) (do=). Falkener 1892 (cd~). Bergholt 1915 (op). Kraitchik 1927 (bl=). These will now eventually be shown on the appropriate history pages.
Magic tours were not included but there are 16 symmetric magic tours, found during the 1849-1888 period, namely those coded 00m and 12a to 12o in my catalogue. The central angles in them are: (bd=) 12m Wenzelides, (bg=) 00m, 12a, 12b Wenzelides, (cd=) 12o Jaenisch, (df=) 12n Jaenisch, (ef=) 12e Wenzelides, (fg=) 12c Beligne, (fn~) 12h Jolivald, 12i, 12j Bouvier, (fo~) 12g Jolivald, (gj=) 12k, 12l Francony, (go~) 12d Jolivald, (no=) 12f Jolivald.