ç Knight's Tour Notes Index
Semi-Magic Tours of Knight on 6×6 Board
By Awani Kumar, Lucknow-226010, INDIA (May 2002).
The following study finds the total number of semi-magic tours on the 6 by 6 board = 88.
Of these: closed magic tours = 26; open magic tours = 62. Further notes are given at the end.
Key: D1=Diagonal sum, top left to bottom right.
D2=Diagonal sum, top right to bottom left.
Magic Constant=111. Mauve band indicates D1 + D2 = 222.
The end-cells, numbered 1 and 36, are marked in blue.
| 1 | 10 | 35 | 28 | 25 | 12 | | 1 | 30 | 13 | 36 | 3 | 28 | | 1 | 30 | 13 | 36 | 7 | 24 | | 1 | 30 | 13 | 36 | 27 | 4 |
| 34 | 29 | 2 | 11 | 8 | 27 | | 14 | 19 | 2 | 29 | 12 | 35 | | 14 | 19 | 8 | 23 | 12 | 35 | | 14 | 19 | 28 | 3 | 12 | 35 |
| 3 | 36 | 9 | 26 | 13 | 24 | | 23 | 8 | 31 | 18 | 27 | 4 | | 29 | 2 | 31 | 18 | 25 | 6 | | 29 | 2 | 31 | 18 | 5 | 26 |
| 30 | 33 | 20 | 5 | 16 | 7 | | 20 | 15 | 22 | 9 | 34 | 11 | | 20 | 15 | 22 | 9 | 34 | 11 | | 20 | 15 | 22 | 9 | 34 | 11 |
| 21 | 4 | 31 | 18 | 23 | 14 | | 7 | 24 | 17 | 32 | 5 | 26 | | 3 | 28 | 17 | 32 | 5 | 26 | | 23 | 8 | 17 | 32 | 25 | 6 |
| 32 | 19 | 22 | 15 | 6 | 17 | | 16 | 21 | 6 | 25 | 10 | 33 | | 16 | 21 | 4 | 27 | 10 | 33 | | 16 | 21 | 24 | 7 | 10 | 33 |
| 1 D1=84 D2=102 | | 2 D1=98 D2=120 | | 3 D1=98 D2=120 | | 4 D1=118 D2=80 |
| 1 | 36 | 9 | 28 | 25 | 12 | | 1 | 36 | 13 | 24 | 33 | 4 | | 1 | 36 | 13 | 24 | 33 | 4 | | 2 | 9 | 12 | 31 | 34 | 23 |
| 34 | 29 | 2 | 11 | 8 | 27 | | 12 | 25 | 34 | 3 | 14 | 23 | | 14 | 25 | 34 | 3 | 12 | 23 | | 11 | 30 | 1 | 24 | 13 | 32 |
| 3 | 10 | 35 | 26 | 13 | 24 | | 35 | 2 | 15 | 22 | 5 | 32 | | 35 | 2 | 15 | 22 | 5 | 32 | | 8 | 3 | 10 | 33 | 22 | 35 |
| 30 | 33 | 20 | 5 | 16 | 7 | | 26 | 11 | 28 | 7 | 18 | 21 | | 26 | 21 | 28 | 17 | 8 | 11 | | 29 | 18 | 5 | 20 | 25 | 14 |
| 21 | 4 | 31 | 18 | 23 | 14 | | 29 | 16 | 9 | 20 | 31 | 6 | | 29 | 16 | 19 | 10 | 31 | 6 | | 4 | 7 | 16 | 27 | 36 | 21 |
| 32 | 19 | 22 | 15 | 6 | 17 | | 10 | 27 | 30 | 17 | 8 | 19 | | 20 | 27 | 30 | 7 | 18 | 9 | | 17 | 28 | 19 | 6 | 15 | 26 |
| 5 D1=110 D2=102 | | 6 D1=98 D2=94 | | 7 D1=98 D2=102 | | 8 D1=124 D2=98 |
| 2 |
9 |
12 |
31 |
34 |
23 |
|
2 |
13 |
4 |
33 |
36 |
23 |
|
2 |
13 |
4 |
33 |
36 |
23 |
|
2 |
13 |
18 |
31 |
36 |
11 |
| 11 |
30 |
1 |
24 |
13 |
32 |
|
5 |
32 |
1 |
24 |
15 |
34 |
|
15 |
32 |
1 |
24 |
5 |
34 |
|
17 |
30 |
1 |
12 |
19 |
32 |
| 8 |
3 |
10 |
33 |
22 |
35 |
|
12 |
3 |
14 |
35 |
22 |
25 |
|
12 |
3 |
14 |
35 |
22 |
25 |
|
14 |
3 |
16 |
33 |
10 |
35 |
| 29 |
18 |
21 |
4 |
25 |
14 |
|
31 |
6 |
29 |
10 |
19 |
16 |
|
31 |
16 |
29 |
20 |
9 |
6 |
|
29 |
6 |
9 |
22 |
25 |
20 |
| 20 |
7 |
16 |
27 |
36 |
5 |
|
28 |
11 |
8 |
17 |
26 |
21 |
|
28 |
11 |
18 |
7 |
26 |
21 |
|
8 |
15 |
4 |
27 |
34 |
23 |
| 17 |
28 |
19 |
6 |
15 |
26 |
|
7 |
30 |
27 |
20 |
9 |
18 |
|
17 |
30 |
27 |
10 |
19 |
8 |
|
5 |
28 |
7 |
24 |
21 |
26 |
| 9 D1=108 D2=114 |
|
10 D1=102 D2=120 |
|
11 D1=102 D2=120 |
|
12 D1=130 D2=92 |
| 2 |
13 |
18 |
31 |
36 |
11 |
|
2 |
15 |
36 |
19 |
22 |
17 |
|
2 |
17 |
8 |
35 |
26 |
23 |
|
2 |
25 |
4 |
33 |
36 |
11 |
| 17 |
30 |
1 |
12 |
19 |
32 |
|
35 |
28 |
3 |
16 |
9 |
20 |
|
7 |
34 |
1 |
24 |
9 |
36 |
|
27 |
32 |
1 |
12 |
5 |
34 |
| 14 |
3 |
16 |
33 |
10 |
35 |
|
14 |
1 |
34 |
21 |
18 |
23 |
|
16 |
3 |
18 |
27 |
22 |
25 |
|
24 |
3 |
26 |
35 |
10 |
13 |
| 29 |
24 |
27 |
4 |
7 |
20 |
|
29 |
4 |
27 |
10 |
33 |
8 |
|
33 |
6 |
31 |
12 |
19 |
10 |
|
31 |
28 |
17 |
8 |
21 |
6 |
| 26 |
15 |
22 |
9 |
34 |
5 |
|
26 |
13 |
6 |
31 |
24 |
11 |
|
30 |
15 |
4 |
21 |
28 |
13 |
|
16 |
23 |
30 |
19 |
14 |
9 |
| 23 |
28 |
25 |
6 |
21 |
8 |
|
5 |
30 |
25 |
12 |
7 |
32 |
|
5 |
32 |
29 |
14 |
11 |
20 |
|
29 |
18 |
15 |
22 |
7 |
20 |
| 13 D1=94 D2=128 |
|
14 D1=130 D2=92 |
|
15 D1=114 D2=110 |
|
16 D1=102 D2=120 |
| 2 |
25 |
28 |
9 |
36 |
11 |
|
2 |
25 |
28 |
21 |
12 |
23 |
|
2 |
25 |
28 |
21 |
12 |
23 |
|
2 |
27 |
18 |
31 |
4 |
29 |
| 27 |
8 |
1 |
12 |
29 |
34 |
|
27 |
10 |
1 |
24 |
29 |
20 |
|
27 |
20 |
1 |
24 |
29 |
10 |
|
17 |
12 |
3 |
28 |
19 |
32 |
| 24 |
3 |
26 |
35 |
10 |
13 |
|
36 |
3 |
26 |
11 |
22 |
13 |
|
36 |
3 |
26 |
11 |
22 |
13 |
|
26 |
1 |
36 |
13 |
30 |
5 |
| 7 |
16 |
5 |
20 |
33 |
30 |
|
9 |
6 |
15 |
32 |
19 |
30 |
|
19 |
16 |
5 |
32 |
9 |
30 |
|
11 |
16 |
9 |
22 |
33 |
20 |
| 4 |
23 |
18 |
31 |
14 |
21 |
|
4 |
35 |
8 |
17 |
14 |
33 |
|
4 |
35 |
18 |
7 |
14 |
33 |
|
8 |
25 |
14 |
35 |
6 |
23 |
| 17 |
6 |
15 |
22 |
19 |
32 |
|
7 |
16 |
5 |
34 |
31 |
18 |
|
17 |
6 |
15 |
34 |
31 |
8 |
|
15 |
10 |
7 |
24 |
21 |
34 |
| 17 D1=102 D2=120 |
|
18 D1=102 D2=120 |
|
19 D1=102 D2=120 |
|
20 D1=112 D2=110 |
| 2 |
33 |
36 |
19 |
4 |
17 |
|
2 |
35 |
24 |
13 |
4 |
33 |
|
2 |
35 |
24 |
13 |
4 |
33 |
|
3 |
16 |
13 |
30 |
27 |
22 |
| 35 |
10 |
3 |
16 |
27 |
20 |
|
23 |
12 |
3 |
34 |
25 |
14 |
|
25 |
12 |
3 |
34 |
23 |
14 |
|
14 |
29 |
2 |
23 |
12 |
31 |
| 32 |
1 |
34 |
21 |
18 |
5 |
|
36 |
1 |
22 |
15 |
32 |
5 |
|
36 |
1 |
22 |
15 |
32 |
5 |
|
17 |
4 |
15 |
28 |
21 |
26 |
| 11 |
22 |
9 |
28 |
15 |
26 |
|
11 |
16 |
9 |
20 |
29 |
26 |
|
11 |
26 |
9 |
30 |
19 |
16 |
|
36 |
7 |
24 |
1 |
32 |
11 |
| 8 |
31 |
24 |
13 |
6 |
29 |
|
8 |
21 |
18 |
27 |
6 |
31 |
|
8 |
21 |
28 |
17 |
6 |
31 |
|
5 |
18 |
9 |
34 |
25 |
20 |
| 23 |
12 |
7 |
30 |
25 |
14 |
|
17 |
10 |
7 |
30 |
19 |
28 |
|
27 |
10 |
7 |
20 |
29 |
18 |
|
8 |
35 |
6 |
19 |
10 |
33 |
| 21 D1=94 D2=128 |
|
22 D1=90 D2=120 |
|
23 D1=90 D2=128 |
|
24 D1=106 D2=112 |
| 3 |
16 |
13 |
30 |
27 |
22 |
|
3 |
28 |
13 |
36 |
5 |
26 |
|
3 |
30 |
13 |
36 |
1 |
28 |
|
4 |
1 |
24 |
13 |
36 |
33 |
| 14 |
31 |
2 |
23 |
12 |
29 |
|
14 |
19 |
4 |
27 |
12 |
35 |
|
14 |
19 |
2 |
29 |
12 |
35 |
|
23 |
12 |
3 |
34 |
25 |
14 |
| 17 |
4 |
15 |
28 |
21 |
26 |
|
29 |
2 |
31 |
18 |
25 |
6 |
|
23 |
4 |
31 |
18 |
27 |
8 |
|
2 |
5 |
22 |
15 |
32 |
35 |
| 32 |
7 |
24 |
1 |
36 |
11 |
|
20 |
15 |
22 |
9 |
34 |
11 |
|
20 |
15 |
22 |
9 |
34 |
11 |
|
11 |
8 |
17 |
28 |
21 |
26 |
| 5 |
18 |
9 |
34 |
25 |
20 |
|
1 |
30 |
17 |
32 |
7 |
24 |
|
5 |
24 |
17 |
32 |
7 |
26 |
|
6 |
29 |
10 |
19 |
16 |
31 |
| 8 |
33 |
6 |
19 |
10 |
35 |
|
16 |
21 |
8 |
23 |
10 |
33 |
|
16 |
21 |
6 |
25 |
10 |
33 |
|
9 |
18 |
7 |
30 |
27 |
20 |
| 25 D1=110 D2=112 |
|
26 D1=102 D2=124 |
|
27 D1=102 D2=120 |
|
28 D1=102 D2=128 |
| 4 |
1 |
24 |
13 |
36 |
33 |
|
4 |
1 |
24 |
13 |
36 |
33 |
|
4 |
1 |
24 |
13 |
36 |
33 |
|
4 |
1 |
36 |
13 |
30 |
27 |
| 23 |
12 |
3 |
34 |
25 |
14 |
|
23 |
14 |
3 |
34 |
25 |
12 |
|
25 |
12 |
3 |
34 |
23 |
14 |
|
35 |
12 |
3 |
28 |
19 |
14 |
| 2 |
5 |
22 |
15 |
32 |
35 |
|
2 |
5 |
22 |
15 |
32 |
35 |
|
2 |
5 |
22 |
15 |
32 |
35 |
|
2 |
5 |
18 |
31 |
26 |
29 |
| 11 |
16 |
9 |
20 |
29 |
26 |
|
21 |
18 |
7 |
28 |
11 |
26 |
|
11 |
26 |
9 |
30 |
19 |
16 |
|
11 |
34 |
9 |
22 |
15 |
20 |
| 6 |
21 |
18 |
27 |
8 |
31 |
|
6 |
29 |
20 |
9 |
16 |
31 |
|
6 |
21 |
28 |
17 |
8 |
31 |
|
6 |
23 |
32 |
17 |
8 |
25 |
| 17 |
10 |
7 |
30 |
19 |
28 |
|
19 |
8 |
17 |
30 |
27 |
10 |
|
27 |
10 |
7 |
20 |
29 |
18 |
|
33 |
10 |
7 |
24 |
21 |
16 |
| 29 D1=94 D2=120 |
|
30 D1=94 D2=128 |
|
31 D1=94 D2=128 |
|
32 D1=80 D2=142 |
| 4 |
13 |
2 |
33 |
36 |
23 |
|
4 |
27 |
8 |
35 |
16 |
21 |
|
4 |
27 |
8 |
35 |
16 |
21 |
|
4 |
27 |
10 |
33 |
16 |
21 |
| 1 |
32 |
5 |
24 |
15 |
34 |
|
9 |
34 |
5 |
20 |
7 |
36 |
|
9 |
36 |
5 |
20 |
7 |
34 |
|
11 |
32 |
5 |
20 |
9 |
34 |
| 12 |
3 |
14 |
35 |
22 |
25 |
|
26 |
3 |
28 |
15 |
22 |
17 |
|
26 |
3 |
28 |
15 |
22 |
17 |
|
26 |
3 |
28 |
15 |
22 |
17 |
| 31 |
6 |
29 |
10 |
19 |
16 |
|
33 |
10 |
19 |
6 |
29 |
14 |
|
29 |
10 |
19 |
6 |
33 |
14 |
|
31 |
12 |
19 |
6 |
35 |
8 |
| 28 |
11 |
8 |
17 |
26 |
21 |
|
2 |
25 |
12 |
31 |
18 |
23 |
|
2 |
25 |
12 |
31 |
18 |
23 |
|
2 |
25 |
14 |
29 |
18 |
23 |
| 7 |
30 |
27 |
20 |
9 |
18 |
|
11 |
32 |
1 |
24 |
13 |
30 |
|
11 |
30 |
1 |
24 |
13 |
32 |
|
13 |
30 |
1 |
24 |
7 |
36 |
| 33 D1=104 D2=120 |
|
34 D1=120 D2=98 |
|
35 D1=124 D2=98 |
|
36 D1=124 D2=102 |
| 4 |
27 |
10 |
33 |
16 |
21 |
|
4 |
27 |
12 |
31 |
16 |
21 |
|
4 |
27 |
12 |
31 |
16 |
21 |
|
4 |
27 |
14 |
29 |
16 |
21 |
| 11 |
34 |
5 |
20 |
9 |
32 |
|
11 |
30 |
5 |
20 |
13 |
32 |
|
11 |
32 |
5 |
20 |
13 |
30 |
|
13 |
30 |
5 |
20 |
7 |
36 |
| 26 |
3 |
28 |
15 |
22 |
17 |
|
26 |
3 |
28 |
15 |
22 |
17 |
|
26 |
3 |
28 |
15 |
22 |
17 |
|
26 |
3 |
28 |
15 |
22 |
17 |
| 35 |
12 |
19 |
6 |
31 |
8 |
|
29 |
10 |
19 |
6 |
33 |
14 |
|
33 |
10 |
19 |
6 |
29 |
14 |
|
31 |
12 |
19 |
6 |
35 |
8 |
| 2 |
25 |
14 |
29 |
18 |
23 |
|
2 |
25 |
8 |
35 |
18 |
23 |
|
2 |
25 |
8 |
35 |
18 |
23 |
|
2 |
25 |
10 |
33 |
18 |
23 |
| 13 |
36 |
1 |
24 |
7 |
30 |
|
9 |
36 |
1 |
24 |
7 |
34 |
|
9 |
34 |
1 |
24 |
7 |
36 |
|
11 |
32 |
1 |
24 |
9 |
34 |
| 37 D1=120 D2=102 |
|
38 D1=120 D2=102 |
|
39 D1=124 D2=102 |
|
40 D1=120 D2=98 |
| 4 |
27 |
14 |
29 |
16 |
21 |
|
4 |
27 |
18 |
31 |
2 |
29 |
|
4 |
27 |
30 |
13 |
16 |
21 |
|
4 |
27 |
30 |
13 |
16 |
21 |
| 13 |
36 |
5 |
20 |
7 |
30 |
|
17 |
12 |
3 |
28 |
19 |
32 |
|
31 |
12 |
5 |
20 |
29 |
14 |
|
31 |
14 |
5 |
20 |
29 |
12 |
| 26 |
3 |
28 |
15 |
22 |
17 |
|
26 |
5 |
36 |
13 |
30 |
1 |
|
26 |
3 |
28 |
15 |
22 |
17 |
|
26 |
3 |
28 |
15 |
22 |
17 |
| 35 |
12 |
19 |
6 |
31 |
8 |
|
11 |
16 |
9 |
22 |
33 |
20 |
|
11 |
32 |
19 |
6 |
35 |
8 |
|
35 |
32 |
19 |
6 |
11 |
8 |
| 2 |
25 |
10 |
33 |
18 |
23 |
|
6 |
25 |
14 |
35 |
8 |
23 |
|
2 |
25 |
34 |
9 |
18 |
23 |
|
2 |
25 |
34 |
9 |
18 |
23 |
| 11 |
34 |
1 |
24 |
9 |
32 |
|
15 |
10 |
7 |
24 |
21 |
34 |
|
33 |
10 |
1 |
24 |
7 |
36 |
|
33 |
36 |
1 |
24 |
7 |
10 |
| 41 D1=124 D2=98 |
|
42 D1=116 D2=110 |
|
43 D1=104 D2=142 |
|
44 D1=80 D2=142 |
| 4 |
33 |
24 |
13 |
2 |
35 |
|
4 |
33 |
24 |
13 |
2 |
35 |
|
4 |
33 |
36 |
19 |
2 |
17 |
|
4 |
35 |
24 |
13 |
2 |
33 |
| 23 |
12 |
3 |
34 |
25 |
14 |
|
23 |
14 |
3 |
34 |
25 |
12 |
|
35 |
10 |
3 |
16 |
27 |
20 |
|
23 |
12 |
3 |
34 |
25 |
14 |
| 32 |
5 |
22 |
15 |
36 |
1 |
|
32 |
5 |
22 |
15 |
36 |
1 |
|
32 |
5 |
34 |
21 |
18 |
1 |
|
36 |
5 |
22 |
15 |
32 |
1 |
| 11 |
8 |
17 |
28 |
21 |
26 |
|
21 |
18 |
7 |
28 |
11 |
26 |
|
11 |
22 |
9 |
28 |
15 |
26 |
|
11 |
8 |
17 |
28 |
21 |
26 |
| 6 |
31 |
10 |
19 |
16 |
29 |
|
6 |
31 |
20 |
9 |
16 |
29 |
|
6 |
31 |
24 |
13 |
8 |
29 |
|
6 |
29 |
10 |
19 |
16 |
31 |
| 9 |
18 |
7 |
30 |
27 |
20 |
|
19 |
8 |
17 |
30 |
27 |
10 |
|
23 |
12 |
7 |
30 |
25 |
14 |
|
9 |
18 |
7 |
30 |
27 |
20 |
| 45 D1=102 D2=132 |
|
46 D1=94 D2=132 |
|
47 D1=98 D2=128 |
|
48 D1=102 D2=128 |
| 4 |
35 |
24 |
13 |
2 |
33 |
|
4 |
35 |
24 |
13 |
2 |
33 |
|
4 |
35 |
24 |
13 |
2 |
33 |
|
5 |
18 |
15 |
22 |
31 |
20 |
| 23 |
12 |
3 |
34 |
25 |
14 |
|
23 |
14 |
3 |
34 |
25 |
12 |
|
25 |
12 |
3 |
34 |
23 |
14 |
|
16 |
23 |
6 |
19 |
14 |
33 |
| 36 |
5 |
22 |
15 |
32 |
1 |
|
36 |
5 |
22 |
15 |
32 |
1 |
|
36 |
5 |
22 |
15 |
32 |
1 |
|
7 |
4 |
17 |
32 |
21 |
30 |
| 11 |
16 |
9 |
20 |
29 |
26 |
|
21 |
18 |
7 |
28 |
11 |
26 |
|
11 |
26 |
9 |
30 |
19 |
16 |
|
24 |
27 |
2 |
11 |
34 |
13 |
| 6 |
21 |
18 |
27 |
8 |
31 |
|
6 |
29 |
20 |
9 |
16 |
31 |
|
6 |
21 |
28 |
17 |
8 |
31 |
|
3 |
8 |
25 |
36 |
29 |
10 |
| 17 |
10 |
7 |
30 |
19 |
28 |
|
19 |
8 |
17 |
30 |
27 |
10 |
|
27 |
10 |
7 |
20 |
29 |
18 |
|
26 |
1 |
28 |
9 |
12 |
35 |
| 49 D1=94 D2=120 |
|
50 D1=94 D2=128 |
|
51 D1=94 D2=128 |
|
52 D1=120 D2=102 |
| 5 |
18 |
15 |
22 |
31 |
20 |
|
5 |
18 |
15 |
22 |
31 |
20 |
|
5 |
18 |
15 |
34 |
7 |
32 |
|
5 |
24 |
13 |
36 |
7 |
26 |
| 16 |
33 |
6 |
19 |
14 |
23 |
|
16 |
33 |
6 |
19 |
14 |
23 |
|
16 |
35 |
6 |
31 |
14 |
9 |
|
14 |
19 |
6 |
25 |
12 |
35 |
| 7 |
4 |
17 |
32 |
21 |
30 |
|
7 |
4 |
17 |
32 |
21 |
30 |
|
19 |
4 |
17 |
8 |
33 |
30 |
|
23 |
4 |
31 |
18 |
27 |
8 |
| 34 |
1 |
28 |
11 |
24 |
13 |
|
34 |
27 |
2 |
11 |
24 |
13 |
|
36 |
27 |
2 |
23 |
10 |
13 |
|
20 |
15 |
22 |
9 |
34 |
11 |
| 3 |
8 |
35 |
26 |
29 |
10 |
|
3 |
8 |
35 |
26 |
29 |
10 |
|
3 |
20 |
25 |
12 |
29 |
22 |
|
3 |
30 |
17 |
32 |
1 |
28 |
| 36 |
27 |
2 |
9 |
12 |
25 |
|
36 |
1 |
28 |
9 |
12 |
25 |
|
26 |
1 |
28 |
21 |
24 |
11 |
|
16 |
21 |
2 |
29 |
10 |
33 |
| 53 D1=120 D2=138 |
|
54 D1=120 D2=112 |
|
55 D1=120 D2=102 |
|
56 D1=98 D2=124 |
| 5 |
28 |
13 |
36 |
3 |
26 |
|
5 |
30 |
3 |
22 |
19 |
32 |
|
5 |
30 |
25 |
12 |
7 |
32 |
|
5 |
32 |
29 |
20 |
7 |
18 |
| 14 |
19 |
4 |
27 |
12 |
35 |
|
28 |
23 |
6 |
31 |
2 |
21 |
|
26 |
13 |
6 |
31 |
24 |
11 |
|
28 |
21 |
6 |
17 |
30 |
9 |
| 29 |
6 |
31 |
18 |
25 |
2 |
|
7 |
4 |
29 |
20 |
33 |
18 |
|
29 |
4 |
27 |
10 |
33 |
8 |
|
33 |
4 |
31 |
8 |
19 |
16 |
| 20 |
15 |
22 |
9 |
34 |
11 |
|
24 |
27 |
14 |
35 |
10 |
1 |
|
14 |
19 |
16 |
3 |
36 |
23 |
|
24 |
27 |
22 |
15 |
10 |
13 |
| 7 |
30 |
17 |
32 |
1 |
24 |
|
15 |
8 |
25 |
12 |
17 |
34 |
|
17 |
28 |
21 |
34 |
9 |
2 |
|
3 |
34 |
25 |
12 |
1 |
36 |
| 16 |
21 |
8 |
23 |
10 |
33 |
|
26 |
13 |
16 |
9 |
36 |
11 |
|
20 |
15 |
18 |
1 |
22 |
35 |
|
26 |
23 |
2 |
35 |
14 |
11 |
| 57 D1=98 D2=124 |
|
58 D1=120 D2=102 |
|
59 D1=92 D2=130 |
|
60 D1=84 D2=138 |
| 5 |
36 |
31 |
12 |
7 |
20 |
|
5 |
36 |
31 |
12 |
7 |
20 |
|
6 |
19 |
8 |
29 |
32 |
17 |
|
6 |
19 |
22 |
15 |
32 |
17 |
| 32 |
13 |
6 |
19 |
30 |
11 |
|
32 |
13 |
6 |
19 |
30 |
11 |
|
9 |
30 |
5 |
18 |
21 |
28 |
|
23 |
30 |
5 |
18 |
21 |
14 |
| 35 |
4 |
33 |
10 |
21 |
8 |
|
35 |
4 |
33 |
10 |
21 |
8 |
|
4 |
7 |
20 |
31 |
16 |
33 |
|
4 |
7 |
20 |
31 |
16 |
33 |
| 14 |
25 |
2 |
23 |
18 |
29 |
|
4 |
25 |
22 |
3 |
18 |
29 |
|
13 |
10 |
15 |
22 |
27 |
24 |
|
27 |
24 |
29 |
8 |
13 |
10 |
| 3 |
34 |
27 |
16 |
9 |
22 |
|
23 |
34 |
27 |
16 |
9 |
2 |
|
36 |
3 |
12 |
25 |
34 |
1 |
|
36 |
3 |
26 |
11 |
34 |
1 |
| 26 |
15 |
24 |
1 |
28 |
17 |
|
26 |
15 |
24 |
1 |
28 |
17 |
|
11 |
14 |
35 |
2 |
23 |
26 |
|
25 |
28 |
35 |
2 |
9 |
12 |
| 61 D1=100 D2=122 |
|
62 D1=80 D2=142 |
|
63 D1=138 D2=98 |
|
64 D1=110 D2=126 |
| 6 |
19 |
22 |
29 |
4 |
31 |
|
6 |
33 |
8 |
15 |
18 |
31 |
|
7 |
24 |
13 |
36 |
5 |
26 |
|
7 |
30 |
13 |
36 |
1 |
24 |
| 21 |
2 |
5 |
32 |
23 |
28 |
|
9 |
14 |
5 |
32 |
35 |
16 |
|
14 |
19 |
6 |
25 |
12 |
35 |
|
14 |
19 |
8 |
23 |
12 |
35 |
| 18 |
7 |
20 |
3 |
30 |
33 |
|
4 |
7 |
34 |
17 |
30 |
19 |
|
23 |
8 |
31 |
18 |
27 |
4 |
|
29 |
6 |
31 |
18 |
25 |
2 |
| 1 |
10 |
35 |
14 |
27 |
24 |
|
13 |
10 |
23 |
2 |
27 |
36 |
|
20 |
15 |
22 |
9 |
34 |
11 |
|
20 |
15 |
22 |
9 |
34 |
11 |
| 8 |
17 |
12 |
25 |
34 |
15 |
|
22 |
3 |
12 |
25 |
20 |
29 |
|
1 |
30 |
17 |
32 |
3 |
28 |
|
5 |
28 |
17 |
32 |
3 |
26 |
| 11 |
36 |
9 |
16 |
13 |
26 |
|
11 |
24 |
21 |
28 |
1 |
26 |
|
16 |
21 |
2 |
29 |
10 |
33 |
|
16 |
21 |
4 |
27 |
10 |
33 |
| 65 D1=102 D2=120 |
|
66 D1=102 D2=120 |
|
67 D1=102 D2=124 |
|
68 D1=102 D2=120 |
| 8 |
19 |
22 |
15 |
30 |
17 |
|
11 |
14 |
35 |
2 |
23 |
26 |
|
11 |
14 |
35 |
2 |
23 |
26 |
|
11 |
16 |
13 |
30 |
9 |
32 |
| 21 |
14 |
7 |
18 |
23 |
28 |
|
34 |
3 |
12 |
25 |
36 |
1 |
|
36 |
3 |
12 |
25 |
34 |
1 |
|
14 |
3 |
10 |
33 |
22 |
29 |
| 6 |
9 |
20 |
29 |
16 |
31 |
|
13 |
10 |
15 |
22 |
27 |
24 |
|
13 |
10 |
15 |
22 |
27 |
24 |
|
17 |
12 |
15 |
28 |
31 |
8 |
| 13 |
34 |
11 |
2 |
27 |
24 |
|
4 |
33 |
6 |
29 |
18 |
21 |
|
4 |
21 |
6 |
17 |
30 |
33 |
|
2 |
27 |
4 |
21 |
34 |
23 |
| 10 |
5 |
36 |
25 |
32 |
3 |
|
9 |
16 |
31 |
20 |
7 |
28 |
|
9 |
16 |
19 |
32 |
7 |
28 |
|
5 |
18 |
25 |
36 |
7 |
20 |
| 35 |
12 |
33 |
4 |
1 |
26 |
|
32 |
5 |
8 |
17 |
30 |
19 |
|
20 |
5 |
8 |
29 |
18 |
31 |
|
26 |
1 |
6 |
19 |
24 |
35 |
| 69 D1=102 D2=120 |
|
70 D1=84 D2=138 |
|
71 D1=84 D2=124 |
|
72 D1=92 D2=130 |
| 11 |
22 |
13 |
36 |
9 |
20 |
|
11 |
22 |
13 |
36 |
9 |
20 |
|
11 |
22 |
31 |
18 |
9 |
20 |
|
11 |
22 |
31 |
18 |
9 |
20 |
| 14 |
3 |
10 |
21 |
28 |
35 |
|
34 |
3 |
10 |
21 |
28 |
15 |
|
16 |
1 |
10 |
21 |
30 |
33 |
|
32 |
1 |
10 |
21 |
30 |
17 |
| 23 |
12 |
15 |
34 |
19 |
8 |
|
23 |
12 |
35 |
14 |
19 |
8 |
|
23 |
12 |
17 |
32 |
19 |
8 |
|
23 |
12 |
33 |
16 |
19 |
8 |
| 2 |
33 |
4 |
27 |
16 |
29 |
|
2 |
33 |
4 |
27 |
16 |
29 |
|
2 |
15 |
4 |
27 |
34 |
29 |
|
2 |
15 |
4 |
27 |
34 |
29 |
| 5 |
24 |
31 |
18 |
7 |
26 |
|
5 |
24 |
31 |
18 |
7 |
26 |
|
5 |
24 |
13 |
36 |
7 |
26 |
|
5 |
24 |
13 |
36 |
7 |
26 |
| 32 |
1 |
6 |
25 |
30 |
17 |
|
32 |
1 |
6 |
25 |
30 |
17 |
|
14 |
3 |
6 |
25 |
28 |
35 |
|
14 |
3 |
6 |
25 |
28 |
35 |
| 73 D1=80 D2=142 |
|
74 D1=100 D2=122 |
|
75 D1=98 D2=124 |
|
76 D1=114 D2=108 |
| 12 |
9 |
2 |
35 |
28 |
25 |
|
14 |
1 |
4 |
33 |
24 |
35 |
|
14 |
1 |
4 |
33 |
24 |
35 |
|
14 |
1 |
4 |
35 |
24 |
33 |
| 1 |
34 |
11 |
26 |
3 |
36 |
|
3 |
22 |
13 |
36 |
5 |
32 |
|
3 |
32 |
13 |
36 |
5 |
22 |
|
3 |
22 |
13 |
32 |
5 |
36 |
| 10 |
13 |
8 |
29 |
24 |
27 |
|
12 |
15 |
2 |
23 |
34 |
25 |
|
12 |
15 |
2 |
23 |
34 |
25 |
|
12 |
15 |
2 |
23 |
34 |
25 |
| 33 |
30 |
17 |
6 |
21 |
4 |
|
21 |
18 |
27 |
8 |
31 |
6 |
|
31 |
28 |
17 |
8 |
21 |
6 |
|
21 |
18 |
27 |
8 |
31 |
6 |
| 14 |
7 |
32 |
19 |
16 |
23 |
|
16 |
11 |
20 |
29 |
26 |
9 |
|
16 |
11 |
30 |
19 |
26 |
9 |
|
16 |
11 |
20 |
29 |
26 |
9 |
| 31 |
18 |
15 |
22 |
5 |
20 |
|
19 |
28 |
17 |
10 |
7 |
30 |
|
29 |
18 |
27 |
10 |
7 |
20 |
|
19 |
28 |
17 |
10 |
7 |
30 |
| 77 D1=96 D2=112 |
|
78 D1=102 D2=120 |
|
79 D1=102 D2=120 |
|
80 D1=102 D2=118 |
| 14 |
9 |
12 |
31 |
16 |
29 |
|
14 |
11 |
2 |
29 |
20 |
35 |
|
14 |
25 |
16 |
9 |
12 |
35 |
|
14 |
25 |
16 |
9 |
12 |
35 |
| 11 |
22 |
15 |
28 |
3 |
32 |
|
1 |
28 |
13 |
36 |
3 |
30 |
|
17 |
8 |
13 |
36 |
27 |
10 |
|
27 |
8 |
13 |
36 |
17 |
10 |
| 8 |
13 |
10 |
33 |
30 |
17 |
|
12 |
15 |
10 |
19 |
34 |
21 |
|
24 |
15 |
26 |
11 |
34 |
1 |
|
24 |
15 |
26 |
11 |
34 |
1 |
| 23 |
34 |
21 |
4 |
27 |
2 |
|
27 |
18 |
25 |
6 |
31 |
4 |
|
7 |
18 |
5 |
22 |
31 |
28 |
|
7 |
28 |
5 |
32 |
21 |
18 |
| 20 |
7 |
36 |
25 |
18 |
5 |
|
24 |
9 |
16 |
33 |
22 |
7 |
|
4 |
23 |
20 |
29 |
2 |
33 |
|
4 |
23 |
30 |
19 |
2 |
33 |
| 35 |
24 |
19 |
6 |
1 |
26 |
|
17 |
26 |
23 |
8 |
5 |
32 |
|
19 |
6 |
3 |
32 |
21 |
30 |
|
29 |
6 |
3 |
22 |
31 |
20 |
| 81 D1=94 D2=128 |
|
82 D1=112 D2=108 |
|
83 D1=102 D2=120 |
|
84 D1=102 D2=120 |
| 14 |
25 |
30 |
7 |
12 |
23 |
|
14 |
25 |
30 |
7 |
12 |
23 |
|
17 |
10 |
7 |
30 |
19 |
28 |
|
18 |
29 |
20 |
7 |
10 |
27 |
| 29 |
6 |
13 |
24 |
31 |
8 |
|
31 |
6 |
13 |
24 |
29 |
8 |
|
8 |
21 |
18 |
27 |
6 |
31 |
|
31 |
6 |
17 |
28 |
21 |
8 |
| 26 |
15 |
28 |
9 |
22 |
11 |
|
26 |
15 |
28 |
9 |
22 |
11 |
|
11 |
16 |
9 |
20 |
29 |
26 |
|
16 |
19 |
30 |
9 |
26 |
11 |
| 5 |
36 |
3 |
16 |
19 |
32 |
|
5 |
32 |
3 |
16 |
19 |
36 |
|
2 |
35 |
22 |
15 |
32 |
5 |
|
5 |
32 |
15 |
22 |
35 |
2 |
| 2 |
27 |
34 |
21 |
10 |
17 |
|
2 |
27 |
34 |
21 |
10 |
17 |
|
23 |
12 |
3 |
34 |
25 |
14 |
|
14 |
23 |
34 |
3 |
12 |
25 |
| 35 |
4 |
1 |
18 |
33 |
20 |
|
33 |
4 |
1 |
18 |
35 |
20 |
|
36 |
1 |
24 |
13 |
4 |
33 |
|
33 |
4 |
13 |
24 |
1 |
36 |
| 85 D1=94 D2=128 |
|
86 D1=94 D2=124 |
|
87 D1=120 D2=124 |
|
88 D1=124 D2=128 |
Further Notes on the 6×6 Semi-magic Tours
added by the editor.
The tours occur in 44 pairs that are reverse numberings of each other, as follows:
1-53, 2-39, 3-36, 4-43, 5-54, 6-88, 7-87, 8-75, 9-76, 10-78, 11-79, 12-72, 13-81, 14-59,
15-82, 16-69, 17-52, 18-83, 19-84, 20-25, 21-85, 22-45, 23-46, 24-42, 26-40, 27-38, 28-29,
30-31, 32-44, 33-80, 34-67, 35-56, 37-68, 41-57, 47-86, 48-49, 50-51, 55-58, 60-70, 61-74,
62-73, 63-71, 64-77, 65-66.
The closed tour 16-69 is one of the quatersymmetric tours, and being symmetric has constant difference
of 18 between numbers in diametrally opposite cells. (This semi-magic property of this quatersymmetric tour was
noted by Maurice Kraitchik in his book on Le Problème du Cavalier 1927, p.36.)
The other twelve pairs of closed tours occur in three groups of four, corresponding to three geometrically
congruent closed tours, numbered from different starting cells:
(a) 1-53, 10-78, 18-83, 65-66;
(b) 11-79, 17-52, 19-84, 55-58;
(c) 12-72, 13-81, 14-59, 21-85;
In case (b), where the underlying tour is piecewise symmetric, there is a constant difference of 6 or 30
between numbers in diametrally opposite cells. (Tour 19 was given by G. P. Jelliss in a note on
‘Piece-Wise Symmetric Tours’ in Journal of Recreational Mathematics Vol.28 No.1 1997 pp.63-64
and Vol.29 No.1 1998, pp.9-71; diagram on p.70)
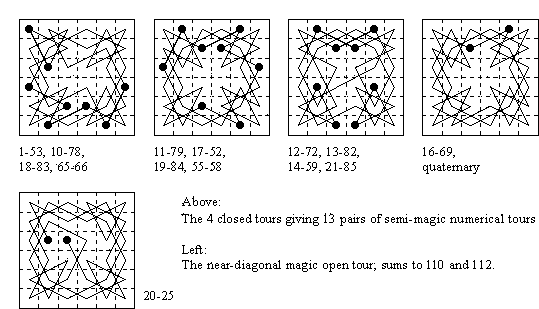
The open tour pair 20-25 is the only one in which the diagonal sums are 111 plus or minus 1, i.e. as near
as possible to the magic constant.
There are 50 tours in which D1 + D2 = 222, consisting of 24 closed 36 open. This group includes all
the closed tours except the pair 1-53.
Back to top

