

by George Jelliss including work by Awani Kumar and Guenter Stertenbrink
This new page started 4 March 2018, updated 22 October 2019 and 21 February 2023.
By oblong we mean rectangular but not square.
The cases listed as unsolved in the previous edition of this page have now been settled.
Sections on this page:
Evenly Even Oblongs (4.h)×(4.k): 4×20, 4×24,
4×28, 8×12.
Oddly Even Oblongs (4.h)×(4.k + 2): 4×18, 4×22,
4×26, 6×12, 6×16, 6×20,
8×10, 8×14, 10×12, 10×16
and 12×14.
It has long been known that magic knight's tours are possible on all (4.h)×(4.k) rectangles 8×8 and larger (e.g. by braid extension of 8×8 magic tours). However the only non-square example that was actually published until recently, as far as we are aware is the 8×12 shown below. It was thought likely that there were also magic tours on 4-rank boards of this type, but none were found until recent work by Awani Kumar (2018) reported below.
Awani Kumar's full results are published online at ArXiv 1802.09340. We give examples here.
He finds magic tours possible on all boards 4×(4.k) with k > 4. There are none on the 4×16 though there are semimagic tours
(magic in the ranks or files only). The numbers of arithmetically distinct magic tours are given as:
88 on 4×20 (XL listing: 4×20) diagrams below
2076 on 4×24 (PDF listing: 4×24) one diagram below
47456 on 4×28 (too many to list here) one diagram below.
All tours on 4-rank boards are asymmetric, so the 88 arithmetically distinct magic tours,
correspond to 44 geometrically distinct, numbered from either end.
Below are diagrams of all 44. The arithmetical forms 45-88 in the XL file are
the reverse numberings of these tours but not in the same sequence,
since they are arranged according to the Frénicle convention.
1-4 and 5-8


9-12 and 13-16
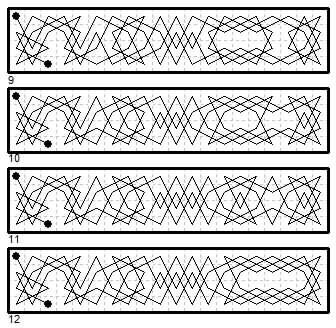

17-20 and 21-24


25-28 and 29-32




One example tour

One example tour

It will be noticed that the end-points and mid-points in all the 4-rank tours always lie within a 3×4 box,
with end-points in the corners, a zebra move apart.
This apppears to be an inherent feature of magic tours on these boards,
and also occurs in examples on larger boards.
This is a simple braid extension of Beverley's tour, given by Jelliss in Variant Chess 1992 (vol.1, issue 8, p.105) just to show it is possible.
01 46 71 76 05 44 67 78 07 42 65 80 72 75 02 45 68 77 06 43 66 79 08 41 47 70 73 04 37 12 83 62 39 10 81 64 74 03 48 69 84 61 38 11 82 63 40 09 49 94 23 28 13 36 59 86 15 34 57 88 24 27 50 93 60 85 14 35 58 87 16 33 95 22 25 52 29 20 91 54 31 18 89 56 26 51 96 21 92 53 30 19 90 55 32 17 | 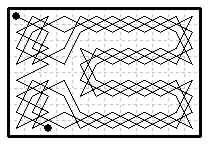
|
In the page on General Theory of Magic Knight Tours (2003) Jelliss gave details of the theorem concerning impossibility of magic knight tours on boards with both sides singly even, which was first published in The Games and Puzzles Journal #25 (online Jan-Feb 2003). He wrote there that: "The above theorems account for all cases except boards (4.m)×(4.n + 2). The question remains, whether a magic knight tour on such a rectangle is possible. I have eliminated the smallest case 4×6 by looking at all the (36) half-tours. The next cases are: 4×10, 4×14, 8×6, 8×10, 8×14, 12×6, 12×10, 12×14, ... Is there an argument to prove the impossibility (if so we can conclude that magic knight's tours are only possible on boards whose sides are both a multiple of 4), or can someone come up with a counter-example?"
This was followed by an Update: In 2011 Jelliss found such counter-examples, 12×14, (now shown at the end of this section). Over the subsequent years many examples have been found on smaller boards. we now show them here in sequence of board sizes.
Awani Kumar has considered this case in his 2018 ArXiv paper cited above
and finds the following numbers of arithmetically distinct magic tours,
which means half these numbers geometrically distinct, since symmetry is impossible.
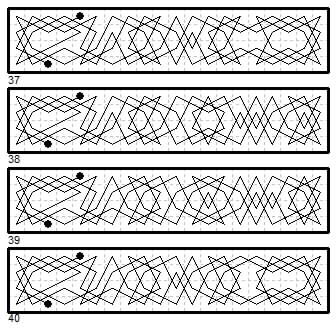
16 on 4×18 See the 8 diagrams below
464 on 4×22 (XL listing: 4×22) one diagram below
9904 on 4×26 (PDF listing: 4×26) one diagram below.
Awani Kumar (2018) finds 16 arithmetically distinct magic tours, numbered 1 to 16,
but there are only 8 geometrically distinct since they occur in pairs that are the reversals of each other
(1=15, 2=16, 3=9, 4=10, 5=11, 6=12, 7=13, 8=14).


diagram of one example

diagram of one example

In the ArXiv paper linked to above Awani Kumar reports finding eight arithmetically distinct magic tours on the 6×12 board,
and over 200 on the 6×16 board, and considers that magic tours are possible on all boards 6×(4.k) with k > 2.
Here are diagrams of the four geometrically distinct magic tours on the 6×12 board found by Kumar.
The first two are also shown in numerical form. Sum of 6-cell lines 219. Sum of 12-cell lines 438.
The righ-hand 6×8 part of the first shows exact axial symmetry.
001 036 067 042 017 020 065 044 015 024 061 046 068 041 034 003 066 043 016 019 064 045 014 025 035 002 037 072 021 018 051 010 023 062 047 060 040 069 004 033 052 055 022 063 050 011 026 013 005 032 071 038 007 030 057 054 009 028 059 048 070 039 006 031 056 053 008 029 058 049 012 027 |
001 036 071 042 063 044 011 028 013 050 059 020 070 041 034 003 010 029 062 045 060 019 014 051 035 002 037 072 043 064 027 012 049 058 021 018 040 069 004 033 030 009 046 061 024 015 052 055 005 032 067 038 007 026 065 048 057 054 017 022 068 039 006 031 066 047 008 025 016 023 056 053 |
a 
| b 
|
The other two tours differ from each other only in the orientation of the 3×4 area that includes the end-points.
c  d
d 
Here are diagrams of the two magic tours on this board that are shown in numerical form in Kumar (2018).
These also have sections 6×12 and 6×8 that show exact axial symmetry.
a  b
b 
Update 22 October 2019: Awani Kumar sends the following further two 6×16 tours.
The first is reentrant and the second has end-points on adjacent cells.
The left 6×8 half of this tour also has exact axial symmetry.
43 88 53 06 59 86 51 02 61 84 23 28 63 82 17 30 54 07 44 87 52 01 60 85 24 27 62 83 16 29 80 65 89 42 05 58 45 50 03 96 71 22 25 76 81 64 31 18 08 55 92 39 04 95 46 49 26 75 72 21 34 15 66 79 41 90 57 10 93 48 37 12 73 70 35 14 77 68 19 32 56 09 40 91 38 11 94 47 36 13 74 69 20 33 78 67 |
17 10 91 76 15 12 93 74 35 40 61 58 33 42 63 56 78 89 16 11 92 75 02 13 72 59 34 41 62 57 32 43 09 18 77 90 01 14 73 94 39 36 71 60 31 44 55 64 88 79 20 07 96 83 24 03 70 49 26 37 66 53 30 45 19 08 81 86 05 22 95 84 25 38 51 68 47 28 65 54 80 87 06 21 82 85 04 23 50 69 48 27 52 67 46 29 |
c 
| d 
|
Update 22 October 2019: Awani Kumar sends the following two 6×20 tours.
The first is reentrant and has the same pattern in the middle 4 files as tour 6×12b above.
This suggests that it could be expanded by braids to 10×16 or 10×20 in the same manner as shown below.
The second has end-points on opposite sides of the board, a {0,5} move apart.
The left 6×12 part of this tour has exact axial symmetry,
and the right 6×8 part is identical to that of tour 6×16d above.
a  b
b 
The question of whether reentrant tours or open tours with different end-point separation than {2,3} are possible has been answered (22 Oct 2019) by the four new tours by Kumar shown above.
Around my 80th Birthday in February 2020, or shortly after, I was sent a series of 80-cell magic knight tours on the 8&time;10 rectangle, consisting of 76 by Guenter Stertenbrink and 24 by Awani Kumar. Apart from a short note in my Jeepyjay Diary pages diagrammed a derived 8×14 tour was diagrammed. Nothing further was published on these results at the time, due to the disruption caused by the Covid pandemic. We now show some examples below.
The total sum 1 + 2 + ... + 79 + 80 = 81 × 40 = 3240.
So 8-cell (column) sum = 3240/10 = 324, and 10-cell (row) sum = 3240/8 = 405.
Four examples by Guenter Stertenbrink
Stertenbrink #1 and #5
27 66 23 50 11 64 01 74 43 46 22 51 26 65 24 49 44 47 02 75 61 28 67 10 63 12 73 04 45 42 52 21 62 25 68 09 48 41 76 03 29 60 19 56 13 72 33 78 05 40 20 53 14 71 18 69 08 39 36 77 59 30 55 16 57 32 37 34 79 06 54 15 58 31 70 17 80 07 38 35 |
02 79 42 39 22 63 20 57 16 65 41 38 01 78 19 60 23 64 25 56 80 03 40 43 62 21 58 17 66 15 37 44 77 04 59 18 61 24 55 26 76 05 36 45 10 71 30 51 14 67 35 46 07 74 31 50 11 70 27 54 06 75 48 33 72 09 52 29 68 13 47 34 73 08 49 32 69 12 53 28 |
 #1 #1
|  #5 #5
|
Stertenbrink #10 and #76
07 74 47 34 09 72 51 30 13 68 46 35 08 73 50 31 12 69 28 53 75 06 33 48 71 10 29 52 67 14 36 45 76 05 32 49 70 11 54 27 77 04 37 44 19 58 21 64 15 66 40 43 80 03 22 61 18 57 26 55 01 78 41 38 59 20 63 24 65 16 42 39 02 79 62 23 60 17 56 25 |
07 74 33 48 09 70 31 52 13 68 46 35 08 73 32 51 10 69 28 53 75 06 47 34 49 30 71 12 67 14 36 45 76 05 72 11 50 29 54 27 77 04 37 44 19 58 21 64 15 66 40 43 80 03 22 61 18 57 26 55 01 78 41 38 59 20 63 24 65 16 42 39 02 79 62 23 60 17 56 25 |
 #10 #10
|  #76 #76
|
Two examples by Awani Kumar
Kumar #1 and #24
03 76 05 72 09 80 39 42 33 46 06 73 02 79 40 49 32 45 36 43 77 04 75 08 71 10 41 38 47 34 74 07 78 01 50 31 48 35 44 37 15 66 17 62 11 70 29 54 23 58 18 63 14 69 30 51 22 57 26 55 67 16 65 20 61 12 53 28 59 24 64 19 68 13 52 21 60 25 56 27 |
05 78 01 72 09 80 39 42 33 46 02 75 04 79 40 49 32 45 36 43 77 06 73 10 71 08 41 38 47 34 74 03 76 07 50 31 48 35 44 37 27 58 25 54 11 70 13 66 17 64 24 55 28 51 30 53 20 63 14 67 59 26 57 22 61 12 69 16 65 18 56 23 60 29 52 21 62 19 68 15 |
 #1 #1
|  #24 #24
|
Some of the 8×10 tours in the Stertenbrink list are closed tours with a complete braid along a short edge. This can be extended, in four different ways, to cover a further four rows forming a magic 8×14 tour. Here is one example that I sent to these correspondents on 18 February 2020, and reported in Jeepyjay Diary for that date. Sum of 1 to 112 = 113 × 56 = 6328, giving 6328/14 = 452, 6328/8 = 791 as the magic constants.
Example 8×14 (Jelliss after Stertenbrink)
07 106 49 64 09 102 47 68 13 100 41 72 17 96 62 51 08 105 48 67 10 101 44 69 16 97 40 73 107 06 63 50 65 46 103 12 99 14 71 42 95 18 52 61 108 05 104 11 66 45 70 43 98 15 74 39 109 04 53 60 27 82 29 88 23 90 35 78 19 94 56 59 112 03 30 85 26 81 34 79 22 91 38 75 01 110 57 54 83 28 87 32 89 24 77 36 93 20 58 55 02 111 86 31 84 25 80 33 92 21 76 37 | 
|
The formations on the fifth to eighth files are familiar: a double Beverley quad, and a Snake-head formation as in the 12×12 magic tour by the Rajah of Mysore.
So far we only have the following two examples. These suggest that solutions exist for all cases k > 2: These are magic tours formed by extending one of Awani Kumar's 6×12 magic tours with a braid. These tours can easily be expanded onto larger boards by extending the braids, e.g. to 12×14, 12×18, 14×16, 16×18, etc.
This was reported, with diagram, in Jeepyjay Diary 24 September 2019. The file and rank sums are 605 and 726 (multiples of 121).
107 072 015 048 105 074 017 046 103 076 019 044 014 049 106 073 016 047 104 075 018 045 102 077 071 108 051 012 069 110 041 022 079 100 043 020 050 013 070 109 052 011 080 099 042 021 078 101 001 060 119 066 111 068 023 040 025 086 095 032 118 065 058 003 010 053 098 081 096 031 026 087 059 002 061 120 067 112 039 024 085 094 033 030 064 117 004 057 054 009 082 097 036 027 088 091 005 056 115 062 007 038 113 084 093 090 029 034 116 063 006 055 114 083 008 037 028 035 092 089 | 
|
This tour was constructed while updating this web-page, October 2019. The file and rank sums are 805 and 1288 (multiples of 161).
069 014 091 148 071 012 089 150 051 032 109 130 053 030 107 132 092 147 070 013 090 149 072 011 110 129 052 031 108 131 054 029 015 068 001 080 159 086 151 088 033 050 035 116 125 042 133 106 146 093 158 085 078 003 010 073 128 111 126 041 036 117 028 055 067 016 079 002 081 160 087 152 049 034 115 124 043 040 105 134 094 145 084 157 004 077 074 009 112 127 046 037 118 121 056 027 017 066 005 076 155 082 007 048 153 114 123 120 039 044 135 104 144 095 156 083 006 075 154 113 008 047 038 045 122 119 026 057 065 018 097 142 063 020 099 140 061 022 101 138 059 024 103 136 096 143 064 019 098 141 062 021 100 139 060 023 102 137 058 025 | 
|
These first-discovered examples of magic tours on oddly even rectangles (Jelliss 2011) were published on the Jeepyjay Diary blog, headed "A Magic Knight Rectangle": Jeepyjay Diary 8 March 2011. We reproduce the text here, with additions:
"Back in 2003 I was able to prove that magic knight's tours were not possible on boards (4.n + 2) by (4.m + 2), but a proof for the (4.n) by (4.m + 2) case eluded me. I now see that that is because there is no such proof! Thanks to a suggestion by John Beasley, that since there is a simple magic king tour on the 2×4 board, a magic knight tour should be possible on a sufficiently large (4.n) by (4.m + 2) board, I looked at the subject again and found two 12×14 examples last night." These are shown below.
These were constructed by the "rolling pin" method that Jelliss devised for 12×12 magic tours. He wrote that "It's surprising I hadn't thought of trying this before. It's just a matter of widening the board." The files add to 1014 = 169×6 and the ranks add to 1183 = 169×7. Each file consists of three pairs adding to 127 and three pairs adding to 211. The ranks are made up of pairs of complements adding to 169. The two tours differ only in a few moves.
141 122 143 038 139 124 127 042 045 030 131 026 047 028 144 037 140 123 128 039 044 125 130 041 046 029 132 025 121 142 035 138 119 126 129 040 043 050 031 134 027 048 036 145 120 063 034 137 014 155 032 135 106 049 024 133 011 064 061 118 013 154 033 136 015 156 051 108 105 158 146 117 012 151 062 059 016 153 110 107 018 157 052 023 065 010 115 060 149 152 111 058 017 020 109 054 159 104 116 147 150 009 114 057 094 075 112 055 160 019 022 053 091 066 007 148 093 074 113 056 095 076 021 162 103 078 006 069 092 073 008 003 082 085 168 161 096 077 100 163 067 090 071 004 083 088 167 002 081 086 165 098 079 102 070 005 068 089 072 001 084 087 166 097 080 101 164 099 |
141 122 143 038 139 124 127 042 045 030 131 026 047 028 144 037 140 123 128 039 044 125 130 041 046 029 132 025 121 142 035 138 119 126 129 040 043 050 031 134 027 048 036 145 120 149 034 137 014 155 032 135 020 049 024 133 011 150 147 118 013 154 033 136 015 156 051 022 019 158 146 117 012 151 148 059 016 153 110 021 018 157 052 023 065 010 115 060 063 152 111 058 017 106 109 054 159 104 116 061 064 009 114 057 094 075 112 055 160 105 108 053 091 066 007 062 093 074 113 056 095 076 107 162 103 078 006 069 092 073 008 003 082 085 168 161 096 077 100 163 067 090 071 004 083 088 167 002 081 086 165 098 079 102 070 005 068 089 072 001 084 087 166 097 080 101 164 099 |
a 
| b 
|