
The following account owes a lot to H.J.R.Murray's chapter on history in his 1951 manuscript The Magic Knight's Tours, a Mathematical Recreation. However, much new information has come to light since he wrote his account. The labels 27a etc, refer to the catalogue of 8 by 8 magic tours on this website, and are not the numbers assigned to the tours by Murray. The squares and diamonds method, described in the previous section of this history, was seen as a possible method of combining the feats of knight's tour and of magic square and von Schinnern (1826) came very close to solving the problem. Contrary to statements in a number of recent books Euler (1759) did NOT construct any magic knight's tours on the 8×8 chessboard (the nearest he came was a 5×5 tour which, because of centrosymmetry, is magic in the four lines through the centre cell).
The first magic knight's tour was composed in 1847 by a certain William Beverley, whose address was given as 9 Upper Terrace, Islington (London). The Dictionary of National Biography (Supplement 1901) has an extensive entry for William Roxby Beverley (born at Richmond, Surrey 1814?, died at Hampstead, London, 17 May 1889) who is probably the knight's tour Beverley, though our evidence for this is purely circumstantial (i.e. he was in the right area of London at the right time). He was a scene painter and designer of theatrical effects, and travelled round the country quite a lot in the course of this work. He is recorded as being in London from 1846 onwards, working at the Princess's Theatre, the Lyceum, Covent Garden and Drury Lane, and exhibited water colours at the Royal Academy. William Roxby Beverley had three older brothers, Samuel, Henry and Robert. Henry Roxby Beverley (1796–1863) controlled the Victoria Theatre, London for a short time, and “died at 26 Russell Square, the house of his brother Mr William Beverley the eminent scene painter”. (This address is currently an Annex of Birkbeck College, University of London.) Their father William Roxby (1765– 1842) was an actor-manager and adopted Beverley as a stage name, after his home town, the old capital of the East Riding of Yorkshire. Upper Terrace no longer exists; it was that part of Upper Street where Islington Town Hall now stands. I could not trace Beverley's name there in the census records for 1851.
Beverley sent the tour first to his friend, the mathematician H. Perigal Jr. of 5 Smith Street, Chelsea, on 5 June 1847, and Perigal sent it on to the editors of the Philosophical Magazine on 29 March 1848 where it was published in the issue for August 1848. The precise addresses and dates cited make one wonder if the publishers were aware of the imminent publication of similar results by Wenzelides in Germany, and were therefore anxious to ensure the priority of the English work.
Perigal's letter reads: “Gentlemen, I inclose for insertion in the Philosophical Magazine a very interesting Magic Square, formed by numbering consecutively the moves of the knight in the grand tour of the chess-board. The knight's march has engaged the ingenuity of many eminent philosophers and mathematicians; but I believe that Mr W. Beverley is the first who has solved the difficult problem of converting it into a magic square. The principle upon which he has effected it, seems to be somewhat akin to that invented by Dr Roget, S.R.S., as explained in his paper on the Knight's Move in vol. xvi of the Philosophical Magazine. Yours very faithfuly, H. Perigal Jun.”
The reverse numbering of any magic tour is magic, and Beverley's tour was presented in both forms, but not with its geometrical diagram as shown here.

As Murray notes, what was most remarkable about this tour was that it did not consist entirely of squares and diamonds but introduced a new type of ‘quarte’ (which we now call a Beverley quarte). This magic tour quickly became widely known. Staunton published the tour in the November number of his Chess Player`s Chronicle 1848 and Hanstein published it in the January number of the Schachzeitung 1849.
It may be noted that the symmetrical right-hand half in Beverley's tour (27a) appears also in von Schinnern's tours (7), (9) and (10). Subsequent composers have shown that this symmetrical path can be replaced by other symmetrical formations, as shown below: by Wenzelides 1849 (27b), Jaenisch 1862 (27cd), Reuss 1883 (27e) and Wihnyk 1885 (27f).

In his 1942 ms Murray claims: “Beverley's tour ... was not discovered by chance but was the fruit of a method based on mathematical analysis which was thought out in advance ... ”. However the analysis he describes, in terms of ‘contraparallel chains’ [see the section on construction of magic tours], is really Murray's own work. I suspect that Beverley found his tour by a more laborious method. Evidence for this is provided by the fact that, when all the tours of ‘quartes’ type (including the new quartes introduced in Beverley's tour) are oriented and arranged in sequence according to Frénicle's method for magic squares, Beverley's tour comes first in the list, and would therefore be the first discovered in a systematic search.
The first magic tour of squares and diamonds type to be found (12a), which is also symmetric, was constructed on 19-20 February 1849 and appeared in the February-March number of the Schachzeitung 1849, under the heading ‘Der Rösselsprung in höchster Kunstvollendung’ {The Knight's Tour in its Highest Perfection}. The author's name is given there only as C...W.. , but later evidence (e.g. the article by Jaenisch in The Chess Monthly 1859) identifies him as Carl Wenzelides.

Murray (1951) describes him as “a pensioned archivist of the Princes of Diedrichstein, who lived in Nicolsburg, Hungary. He was an invalid, confined for many years to his couch, who found a welcome relief to the tedium of his life in the composition of chess problems and knight's tours. He has told the story of his research on the knight's problem in two most human and interesting articles in the Schachzeitung, the first completed on 1 October 1848 (Sztg, IV, 41 and 242) the second completed on 25 March 1850 (Sztg, V, 212, 230). Extracts from his correspondence with Hanstein are [also] given in the Schachzeitung XIII, 174.”
Donald Knuth wrote to me 24 May 1994 as follows: “I see that Ahrens and Rouse Ball give the spelling Wenzelides, as in the German Schachzeitung. But de Jaenisch says Vencélidès was Hungarian; de Jaenisch was in correspondence with Vencélidès [shortly] before the latter's death. I found Karl Wenzelides, 'polyhistorian', listed in Biographisches Lexikon des Kaiserthums Österreich by Wurzbach, 1856-1891; this is almost certainly our man! Born September 1770 in Troppau (now Opava in the Czech Republic), died 6 May 1852 in Nikolsburg (now Mikulov). He wrote poetry and music, besides works on the Bronze Age, etc; many of his books and letters were in the Troppauer Museum.”
In all Wenzelides obtained seven symmetrical magic tours (stated in Schachzeitung 1850, p.238), but owing to his death, and that of the editor Hanstein, two were lost. The last three might never have been published but for the insistence of Jaenisch to the then editor O. von Oppen (Schachzeitung May 1858). The five published were 12a, 12b, 12e, 12m, 00m. Wenzelides also produced a variant of Beverley's tour (27b). The tours 12a and 12b have the extra property that the sum of the two diagonals is 520 (twice the magic constant).
Among the 16 symmetric magic tours now known, 00m is still the only cyclic example; that is, it gives two distinct arithmetical forms related by a cyclic shift of the numbering. The numbering shown on the right here is related to that on the left by adding 16 or subtracting 48, i.e. a cyclic shift of 16 cells.

Murray notes some other lost work: “In the same year (1849) the Schachzeitung announced that A.F.Svanberg, Professor of Mathematics in the Stockholm University, had also discovered four magic tours ‘as a result of mathematical reasoning’, but these were never published and are not to be found among Svanberg's papers now preserved in Stockholm. All that we know of them is that they were found later than Wenzelides's first tour and that one was ‘concordant’ with Wenzelides's first tour, whatever that may mean.”
Mysore was annexed by the British following the death of Tippoo at Seringapatam 1799. What happened next is told in The Golden Book of India by Sir Roper Lethbridge, 1893, pp.362-8; in summary: The British resolved that Mysore revert to control of the family of its ancient rulers. An infant son of Chamraj, by name Krishnaraj, was placed on the gadi. During the minority of the Maharaja Krishnaraj 1799–1810 the state was administered by a Diwan or Prime Minister, the famous Purnaiya. The affairs of the state however fell into disorder after the retirement of Purnaiya; and the ‘misgovernment’ of the Maharaja was terminated by the British Government assuming the direct administration in 1831, retaining the Maharaja as titular sovereign. On the 18th June 1865 the Maharaja adopted as his son and successor the young prince Maharaja Chama Rajendra Wadiar, whose adoption was sanctioned in 1867. Maharaja Krishnaraj died in 1868. The rulers of Mysore were called Wadiar or Wodeyar, being a plural or honorific form of Odeya, Kanarese for ‘Lord’. Assuming the age of majority in India was 21 would give the date of birth of Krishnaraj as 1790.
Thus it would seem Krishnaraj had more leisure to pursue his own interests from 1831 to 1868, and one of these was the investigation of knight's tours. These tours were preserved in a manuscript by Pandit Harikrishna Sharma Jyotishacharya, written in 1871, which was printed in Devanagari script by Venkateshwar Steam Press, Bombay in 1900, and is reproduced, with English commentary, in the book Indian Chess by S.R.Iyer (NAG Publishers, Delhi 1982). The English commentary in Section IX reads: “Now some ways of horse-movement are being explained ... some of those ways mentioned by the King of Karnataka, H. H. Shri Krishna Udayar, are quoted below.” Karnataka is the modern name (since 1973) for Mysore, so the King of Karnataka may be identified with the Rajah of Mysore. This manuscript contains one 8 by 8 magic knight's tour. This tour was independently discovered by Francony in 1881, and appeared in N. Rangiah Naidu's Feats of Chess 1922 without mention of its author. This 8×8 magic tour is #37 among the 82 tour diagrams given in Indian Chess. It is a closed tour of squares and diamonds type, and is fourfold magic, in that it can be numbered magically from four different origins by cyclic shift of the numbering (i..e from f5, d1, b7 as well as f3).
Murray wrote: “It was not known in Europe until 1938 that Indian players had also busied themselves with magic tours and that a closed unsymmetrical magic tour had been discovered in Mysore on 31 July 1852. A contemporary silk handkerchief bearing this tour, which it ascribed to Maharajah Kristna Rajah Wodayer Bahaudah, the Rajah of Mysore, was exhibited at the Margate Easter Chess Congress, 1938.”
Another sighting of this silk was reported by Major J. Akenhead in a letter dated 12 March 1947 to Fairy Chess Review, vol. 6, April 1947, p.84: “I was in Mr A. Hammond's (Emil, Burlington Gardens) yesterday and found that he had a piece of silk framed on which was a magic knight-tour invented, as the wording stated by Maha Rajah Kristna Rajah Wodaye, Behauder Rajah of Mysore, on 31st July 1852.” The present whereabouts of the silk (or silks?) is unknown.*
* Update! On 20 July 2013 I received the following email message from Jon Crumiller of Princeton, New Jersey, USA: "Actually the whereabouts are known, because I am the proud owner of that silk. Attached is a photo. The silk is currently on display (through September 15) at the World Chess Hall of Fame in St. Louis. A photo and description of the silk are near the bottom of that webpage." Alongside is the image enclosed with the email (click to see a larger version).
Of even greater interest is the inclusion of a 12×12 magic knight's tour, diagram #3, also formed on the squares and diamonds principle. This is the earliest known magic knight's tour on a board larger than the 8×8. The existence of this tour was not known to Murray. No other magic tour on a larger board is known until 1885, when Wihnyk gave a 16×16 example, and no other on the 12×12 is known before 1932 when E. Lange added a border to an 8×8 tour. The snake-like pattern of this tour is similar to that of tour (11) by von Schinnern, and one wonders if von Schinnern's little book was in the Rajah's library.

Carl Friedrich Andreyevich Jaenisch (1813–1872) was from St Petersburg and like that city his name is given in different forms: German 'von J', French 'de J', or with neither prefix, or sometimes in a different transliteration from the Cyrillic as 'Yanich'. His symmetric magic tours of quartes type, 12n and 12o were first published in Chess Monthly 1859 in an article previewing his three-volume Traité des Applications de l'Analyse mathématiques au Jeu des Echecs, 1862. This contained a further four magic tours: 27c and 27d of Beverley type, 00a and 00e of cyclic type. Tour 00a has diametrally opposite cells differing by 8.
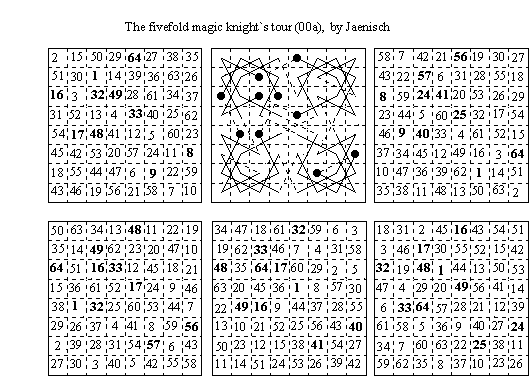
The tour 00a is especially interesting since it is magic when numbered from five different origins, the most ever achieved. The geometrical form and all five numberings are shown above. (There are of course a further five numberings by reversing all these five, numbering in the opposite direction. Also, of course, each square can be presented in eight different orientations by rotation and reflection.)
Murray wrote: “Jaenisch's contemporaries regarded his treatment of magic tours as definitive, and no new magic tours were discovered during the next fourteen years. Then in 1876 Charles Bouvier, a Frenchman, published a magic tour (05a) which struck new ground in containing two pairs of irregular quartes, and Dr Exner published a memoir, Der Rösselsprung als Zauber-quadrat, {The knight's tour as magic square} which contained fifteen magic tours, of which three (27i, 34a, 34e) were new and another gave a fifth arithmetical version of Jaenisch's (00a). Parmentier (Complément 5, note**) says that Exner's memoir gives an ingenious but very laborious method of construction with consecutive quartes in the same quadrant (which Wenzelides called double-quartes). E.C.Caldwell contributed a new magic tour (05b) to the English Mechanic in 1879.”
“A period of great activity in the composition of magic tours opened in France in 1880, largely stimulated by M.A.Feisthamel in his chess column in Le Siècle {The Age} 1876–1885, in which he published all the known magic tours and new ones as they were produced. Two new tours appeared in 1880, six in 1881, fourteen in 1882, thirty-nine in 1883 and two in 1884. The contributors of these tours all adopted pseudonyms: A = Béligne (2 tours); Adsum = C. Bouvier (4 tours); Célina = E. Francony (11 tours); F = Feisthamel (1 tour); Paul de Hijo = Abbé Jolivald (4 tours); Palamède = Ct Ligondès of Orleans (31 tours); X à Belfort = Prof C. E. Reuss (7 tours). Altogether this group added 63 geometrical and 80 arithmetical magic tours. The majority are composed of quartes, but they also include tours in which no use is made of quartes. The first of these was contributed by Charles Bouvier in 1882. None of these composers gave any indication of the methods used to obtain his tours.”

“M. Wihnyk of Frauenburg, Kurland, contributed two articles on magic tours to the Schachzeitung in
1885 (pages 98 and 289). The first gave a modification of Beverley's tour in which irregular quartes were employed,
in order to correct Wenzelides's statement that irregular quartes could not be used in magic tours (but which
Charles Bouvier had done in 1876). The second article showed how Beverley's tour could be extended to give magic
tours on all boards of 4n×4n with (n > 2) cells, and thus opened a new field for explorers.” [As we
noted above Murray was not aware of the 12×12 magic knight tour constructed by the Rajah of Mysore between
1852 and 1868.] Here is Wihnyk's tour in numerical form:
234 87 152 41 232 89 154 39 218 103 168 25 216 105 170 23
151 42 233 88 153 40 231 90 167 26 217 104 169 24 215 106
86 235 44 149 92 229 38 155 102 219 28 165 108 213 22 171
43 150 85 236 37 156 91 230 27 166 101 220 21 172 107 214
238 83 148 45 228 93 160 33 224 97 164 29 212 109 174 19
147 46 237 84 157 36 225 96 161 32 221 100 173 20 211 110
82 239 48 145 94 227 34 159 98 223 30 163 112 209 18 175
47 146 81 240 35 158 95 226 31 162 99 222 17 176 111 210
242 79 144 49 66 255 64 129 194 127 192 1 208 113 178 15
143 50 241 80 131 62 67 254 3 190 195 126 177 16 207 114
78 243 52 141 256 65 130 63 128 193 2 191 116 205 14 179
51 142 77 244 61 132 253 68 189 4 125 196 13 180 115 206
246 75 140 53 252 69 134 59 198 123 188 5 204 117 182 11
139 54 245 76 133 60 251 70 187 6 197 124 181 12 203 118
74 247 56 137 72 249 58 135 122 199 8 185 120 201 10 183
55 138 73 248 57 136 71 250 7 186 121 200 9 184 119 202
Diagonal sums: odd = 1898, even =2090. For the geometrical diagram see the page on 16×16 magic tours.
Murray's account continues: “The period of activity in France ended with General Parmentier's paper on the Knight's Problem which was read at the Marseilles meeting (1891) of the French Association pour l'Avancement des Sciences, which he supplemented at the Pau meeting in 1892. These papers were subsequently published with diagrams of 83 geometrical and 110 arithmetical solutions of magic tours. This remained the standard collection of magic tours for forty years.”
Murray's History continues: “Although four new solutions were published in French chess columns between 1892 and 1912 (three by Ct Ligondès and one by Grossetaite) the main interest of French composers turned to the construction of magic two-knight's tours in quaternary symmetry. Ct Ligondès (who had previously printed privately his magic tours as he discovered them) collected all the magic two-knight's tours in quaternary symmetry in a privately printed work in 1911. This work contained all the 292 solutions to this facet of the knight's problem.” [However, a copy of a cutting sent to me by D.E.Knuth, which has been traced to the chess column conducted by ‘E.H.’ in the Glasgow Weekly Herald for 1874, contains a magic two-knight tour, and I have found several others in the same source, so it seems much work was done on this subject earlier. This type of tour is not really a knight's tour but an empress (knight + rook) or emperor (knight + wazir) tour, since it consists of two congruent knight paths (one the reflection of the other) connected by a rook move.]
Murray contines: “M.B.Lehmann, in the fourth edition of his Neue mathematische Spiele, 1932, included geometrical diagrams of all the known magic tours on the chessboard, and a selection of tours on larger boards which had been composed by E.Lange of Hamburg by adding a frame to the chessboard and using suitable parent tours. Subsequently Lehmann found a new magic tour which he published in Le Sphinx, August 1933. This brought the number of geometrical magic tours on the chessboard to 88.”
This was the position when Murray began to study magic knight's tours in the summer of 1935. By analysing Beverley's tour Murray formulated his idea of ‘contiguous contraparallel chains’ and found all 14 tours of this type, writing: “The analysis was carried out by me on July 18 and 20, 1935 and was the first fruit of my examination of magic tours, and was given in summary in the Fairy Chess Supplement to The Problemist for February 1936.” He later found that only four of these were new, not twelve as he had supposed. “It was not until January 1939 that I succeeded in obtaining M.B.Lehmann's Neue Mathematische Spiele (1932), which included all the magic tours on the chessboard that were then known. By this time I had perfected my method of constructing magic quarte-tours, and had already obtained 49 of the 87 tours in Lehmann's work. By 1941 I had independently discovered by my methods all the tours given by Lehmann and brought up the number of geometrical solutions to 96, of which eight were entirely new [including the four mentioned above].” His methods are described in the Magic Tours section. His unpublished manuscript The Magic Knight's Tour, a Mathematical Recreation (1951) included a complete catalogue of the 8×8 tours, including the eight he found himself, and both forward and reverse numberings. His numbering scheme for the tours is based on the underlying structure of the tours in terms of quartes. In this ms Murray also extended his methods to larger boards, including a method of Extended Quartes which he had described in Fairy Chess Review August 1942. Murray died in 1955 at the age of 87 without having published a book on tours. Many of his papers on the subject (26 boxes) were deposited at the Bodleian Library Oxford at the time of his death, but three manuscripts (1930, 1942, 1951) were retained by his family until deposited at the Bodleian in 1991.
Shortly after Murray's death his method of extended quartes was adapted by H.E. de Vasa (who was at the time apparently an invalid living in Paris) in correspondence with T.H. Willcocks to construct the first diagonally magic knight's tours (i.e. with the two main diagonals also adding to the magic constant) on boards 16×16 and larger. Three by de Vasa were eventually published in 1962 and one by Willcocks in 1968. Willcocks subsequently constructed some 12×12 tours that were magic in one diagonal.
After studying Murray's 26 boxes of papers in the Bodleian Library the present writer, G.P. Jelliss, produced a special issue of Chessics (vol.2 no.26 Summer 1986) on ‘Magic Tours’ which included a catalogue of all the tours known to Murray, but classified in a different manner. Each tour was given a two-digit code based on the separation of its end-points: closed tours being coded 12 and cyclic closed tours 00. (One tour diagram 03b given in the catalogue was not in fact magic.) The description of the methods of construction given in Chessics concluded “It should be possible to apply modern computer methods to ascertain whether all the magic 8×8 knight tours of this type [i.e. using regular quartes] have been discovered.”
This project was immediately taken up by T.W. Marlow who found five new tours which were published in the January 1988 issue of The Problemist. This work has several times been independently confirmed using more powerful computer methods, first by Michael Gilpin of Michigan Technological University, USA (1997).
In 1991 GPJ discovered the continued existence of the further Murray manuscripts (including the 1951 catalogue). Details of these, particularly the history of magic tours as recounted here, were published in The Games and Puzzles Journal and subsequently on this website. I also devised new methods of constructing 12×12 and 16×16 magic tours (none proved to be magic in the diagonals), and most recently (2003) proved the impossibility of magic knight's tours on rectangles 4m + 2 by 4n + 2.
In January 2003, after encountering the catalogue of magic tours on the Knight's Tour Notes site, Tim Roberts of Central Queensland University, Australia (2003), devised a program to search for new magic tours of irregular type and discovered two new magic tours. These were published online in The Games and Puzzles Journal, issue 25.
In March 2003 Awani Kumar (India) solved the long-standing problem of a diagonally magic tour on the 12×12 board, obtaining four examples. These were also published online in The Games and Puzzles Journal, issue 26.
At the beginning of 2003 I was contacted by Günther Stertenbrink (Germany) who had a plan for searching for magic knight's tours using distributed programming. Not knowing anything about this, and no actual tours being shown, I was skeptical, but subsequently his plan was developed into an actual program by Jean-Charles Meyrignac (France) and was run simultaneously on a number of computers, principally by Hugues Mackay (Canada). This international project, which involved a separate search for each of the 136 distinct possible positions for the end-cells of the tour, was carried out during June - August 2003, and found a further five (geometricaly distinct) magic tours. For technical details go to: Computing Magic Knight Tours. This project also regenerated all the existing known tours. So it now seems safe to say that the chapter on 8×8 magic knight's tours has at last been brought to a conclusion, and there are no more to be found. This achievement was enough to have been given a headline in MathWorld, though this emphasised the negative aspect, that none of the tours is magic in the diagonals, rather than the positive side of the completion of a task that has occupied a whole army of researchers for over 150 years.
The following is a list of the 8×8 magic knight tours in historical sequence of discovery or publication together with the numbers given to them in various catalogues. P = Parmentier (1891), L = Lehmann (1932), M = Murray (1951), J = Jelliss (1986). The most recently discovered tours are coded according to the 1986 method, which allowed for new discoveries to be interpolated.
| 1. W. Beverley Philosophical Magazine (April 1848) p.102 | P12 L592 M17 J27a | |||
| 2-7. C. Wenzelides Schachzeitung (1849) p.247 Fig.107 (1850?) (1858) p.274 | P8 L547 M35 J12a P11 L591 M7 J27b | P2 L550 M8 J12e P7 L546 M34 J12b | P5 L552 M36 J12m P61 L539 M64 J00m | |
| 8. Rajah of Mysore (1852) | P21 L559 M50 J00b and P97-99 | |||
| 9-10. C. F. de Jaenisch Chess Monthly (1859) | P4 L540 M76 J12n | P3 L548 M73 J12o | ||
| 11-14. C. F. de Jaenisch Traite des Applications de l'Analyse Mathematiques au jeu des Echecs (1862) |
P1 L561 M75 J00a and P88-90, 110 P27 L562 M62 J00e and P81-83 | P13 L587 M28 J27c P14 L588 M31 J27d | ||
| 15-17. Exner Der Rosselsprung als Zauber-quadrat (1876) | P42 L583 M70 J27i | P40 L605 M24 J34a | P41 L573 M74 J34e | |
| 18. C. Bouvier (1876) | P25 L580 M48 J05a | |||
| 19. E. C. Caldwell English Mechanic (1879) | P18 L578 M54 J05b | |||
| 20. Beligne (1880) | P28 L548 M86 J12c | |||
| 21. Anonymous (1880/1881) | P29 L567 M33 J00i | |||
| 22. Beligne (1881) | P34 L604 M29 J27j | |||
| 23-26. E. Francony (1881) | P19 L557 M53 J00c and P94-96 P26 L572 M37 J05f | P30 L599 M30 J27k P10 L600 M26 J27l | ||
| 27-29. C. Bouvier (1882) | P56 L584 M65 J05d | P77 L545 M93 J12i | P78 L544 M91 J12j | |
| 30-33. P. Jolivald (1882) | P31 L554 M84 J12d P30 L541 M82 J12f | P32 L551 M88 J12g P33 L553 M85 J12h | ||
| 34-39. E. Francony (1882) | P22 L579 M59 J05c P17 L577 M47 J05e | P80 L542 M94 J12k P82 L543 M92 J12l | P20 L582 M51 J23a P35 L603 M27 J27m | |
| 40-41. Ligondes (1882) | P43 L606 M10 J14a | P44 L607 M3 J14b | ||
| 42-71. Ligondes (1883) | P53 L564 M43 J00f and P104-106 P66 L566 M46 J00g and P107-108 P75 L628 M41 J01a P65 L634 M42 J01b P68 L635 M61 J01c P73 L575 M52 J03a P76 L629 M38 J03c P49 L624 M39 J03d P69 L618 M63 J03e P64 L619 M49 J03f | P36 L570 M66 J05g P67 L586 M60 J14c P57 L636 M67 J23c P54 L625 M6 J23d P51, 91 L622 M9 J23e P52 L623 M14 J23f P49 L624 M2 J23g P50 L621 M18 J23h P48 L615 M16 J23i P47 L616 M11 J23j | P46 L617 M19 J23k P45 L614 M4 J23l P58 L612 M56 J23m P59 L613 M55 J23n P55 L620 M15 J25a P38 L632 M23 J34b P37 L633 M25 J34c P39 L604 M22 J34d P70 L585 M69 J34f P76 L629 M71 J34g | |
| 72-78. Reuss (1883) | P23 L558 M44 J00d and P100-102 P24 L560 M45 J00j and P103 P15 L589 M89 J7e | P62 L608 M5 J27p P61 L609 M12 J27q P63 L610 M13 J27r | P60 L611 M20 J27s | |
| 79. E. Francony (1883) | P81 L563 M96 J00k and P85 | |||
| 80. C. Bouvier (1883) | P79 L565 M95 J00l and P86 | |||
| 81. Ligondes (1884) | P83 L626 M40 J23b | |||
| 82. Feisthamel (1884) | P71 L630 M68 J14d | |||
| 83. M. Wihnyk Schachzeitung (1885) p.98 | P16 L590 M32 J27f | |||
| 84. Grossetaite (1896) | L574 M90 J01e | |||
| 85-87. Ligondes La Mode du Petit Journal (1906) (1910) (1911) | L568 M1 J00h | L595 M83 J23o | L597 M87 J23p | |
| 88. M. B. Lehmann Sphinx August 1933 | M81 J16a | |||
| 89-96. H. J. R. Murray Fairy Chess Review (February April August 1936) (November 1939) (1940) | M78 J27g M77 J27h M80 J27n | M79 J27o
M57 J01d M21 J01f? | M58 J12p M72 J03g | |
| 97-101. T. W. Marlow The Problemist (January 1988) | J01g J01h | J03b J23q | J25b | |
| 102-103. T. S. Roberts The Games and Puzzles Journal (January 2003) | J01i | J14e | ||
| 104-108. H. Mackay, J-C. Meyrignac and G. Stertenbrink Knight's Tour Notes (June - August 2003) | J00n J00o | J14f J27t | J07a |
Note: the total 108 is the number of geometrically distinct magic tours. Some of these are closed tours that can be numbered from various different starting points; specifying the terminals increases the count to 140. Each of these paths can then be numbered from either end giving 280 distinct arithmetical magic tours. Finally each of these arithmetical magic tours can be oriented in eight different ways by rotation and reflection, giving a total of 2240 magic numerical arrays (matrices).