
The description 'squares and diamonds' is applied to the octonary pseudotour consisting of four 4-move circuits that can be drawn to fill a 4×4 area, as in the central area in Collini's method. In retrospect Rudrata's 4×8 tour and several of the other mediaeval 4×8 tours can be seen to use squares and diamonds at one end, so the germ of the idea was about for a long time before it took hold. It should be noted that I apply the term squares and diamonds method strictly to tours formed from the 4-move circuits by minimal deletions; the term is not applicable if more than one move in any circuit is deleted. I also use the shorter term rhombic method on occasion.
Tours of the 8×8 chessboard of rhombic type first appeared in 1825, and the method has a special place in the history of the search for a simple systematic method of construction. It probably led Roget to his independent method of quickly constructing a tour between given squares, 1840, and was important in leading towards the construction of the first magic knight's tours in 1848.
It is necessary to correct a number of errors in some accounts of the history, particularly in the attribution of the invention of the squares and diamonds idea to undeserving sources and the confusion of the method with Roget's method which is an important related but independent method.
The first published tours of rhombic type appear to be two given by 'F.P.H.' (full name unknown) in an appendix to the 6th edition (1825) of the Studies of Chess. This is an English edition (usually attributed to Peter Pratt) of L'analyse du jeu des Echecs by the great chess player François-André Danican Philidor (1726–95), which first appeared in 1749 and in English in 1750. F.P.H. gives two tours showing fixed differences of 16 and 8 between diametrally opposite cells. The tours with difference 16 and 8 are also stipulated to satisfy the condition: “If the board were divided into quarters ... the difference between any two squares through whose centres a line drawn would cut that quarter in half, to be 2.” This numerical condition is equivalent to the geometrical one that the quarters be formed on the squares and diamonds plan.

The first full exposition of the 'squares and diamonds' method was given in a curious little 36-page booklet by C. R. R. von Schinnern, Ein Dutzend mathematischer Betrachtungen {A dozen mathematical contemplations} Geistinger, Vienna, 1826 with preface dated 1825, [a photocopy of this booklet, with commentary on the other mathematical items, was made available to me by Professor D. Singmaster]. The article (pages 16–29 of the booklet) has the title: “Die Formeln für den geometrisch-aritmetischen Rösselsprung”. The formulae referred to indicate the sequence in which the quarters are visited and whether the moves within them follow a square (quadrat) or diamond (rhombus) path.
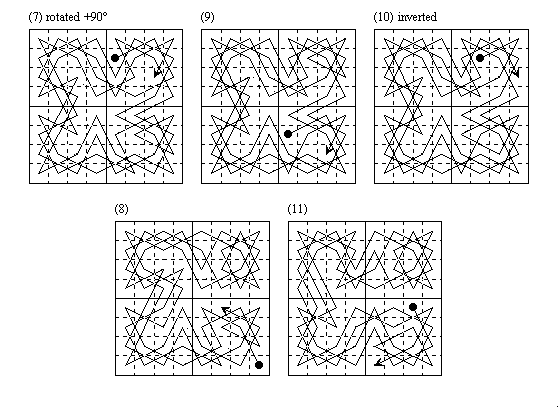
Although there are 8 tour diagrams (presented in numerical form) there are only 4 geometrically distinct tours, the others being reflections or reversals, or in one case a cyclic renumbering. All four tours are semi-magic in Murray's sense, i.e. their files or ranks all add to 260 but not both. I show the tours here in geometrical form, oriented so that the files are magic. Two, (8) and (11), are open and two, (7) and (9), are closed; another closed tour (10) is a numerical variation of (9), but the same geometrically. Tour (10) is the same as (9) but numbered from f7 to h6.
The tour (11) is particularly notable, since it has 8 files and 6 ranks magic. The first and fourth ranks add to 260±4. It is impossible to get any closer than this to constructing a magic tour, short of the real thing, which took another 21 years!
This work of von Schinnern unfortunately does not seem to have gained proper recognition, and the squares and diamonds method has been undeservedly misattributed to later authors, particularly Ciccolini or Roget.

In his book Del Cavallo degli Scacchi (1836) Ciccolini gave the diagram of the 16 four-move circuits and one closed tour of squares and diamonds type, as shown in the inset diagram, but went on to derive other tours from it by Euler's method, which destroys the squares and diamonds structure. Ciccolini's purpose (if my interpretation of the Italian is correct) was to solve the Collini problem of finding a route between any two squares of opposite colour by providing a complete catalogue of such tours. For example the first tour derived from the master tour is presented as 1–25, 64–43, 26–42, indicating that one follows the given tour as far as cell 25 (f4) then instead of 26 we go to 64 (e6) then back to 43 (c1) and from there to 26 (e2), ending at 42 (a2). This catalogue seems to have been misinterpreted as a list of squares and diamonds tours by Q. Poirson-Prugnaux in his Introduction pratique du jeu des echecs (1849), and from thence it was cited as such in the influential collections of mathematical recreations by Lucas (1895) and Ahrens (1901), neither of whom had seen the original.
The project of constructing tours of squares and diamonds that solve the Collini problem was in fact carried out by J. E. Thomas de Lavernède, 1839 (Thomas appears to be his surname). He calls the elements 'quarrés' and 'losanges'. I give only his first example. He gives a laborious list of 2048 tours. This number arises since there are 64 choices of the initial square and 32 of the final square (of the other colour), thus giving 64×32 = 2048 cases.
However, the task is far simpler than this figure suggests, since the number of geometrically distinct ways of placing the two end-points is only 136. This total seems to have been first correctly counted, after several abortive attempts by others, by Heinrich Meyer in Leisure Hour (1873).
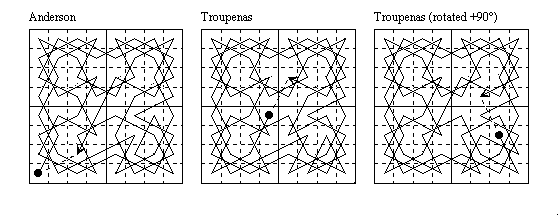
The squares and diamonds method was also expounded briefly, with explanations and example tours, by E. Troupenas in the early chess magazine Le Palamède in 1842. His work was stimulated by an example sent to him from England by a certain Mr Anderson. Since this tour is started in a corner it is not strictly squares and diamonds, though it would be if numbered from a different origin, but it is Rogetian (as described in the last section below). [It is tempting to wonder if its composer could have been John Henry Anderson (1815–1874) known as 'The Wizard of the North', an active stage magician and impresario of the time, who may perhaps have exhibited the knight's tour using Roget's method? But this is only fanciful conjecture on my part, alas.] The two examples by Troupenas, were given wider circulation in Aaron Alexandre's influential collection of chess problems, Beauties of Chess, published simultaneously in French, German and English editions in 1846. The first Troupenas tour is quoted by Rouse Ball (1939) misleadingly as 'Roget's Solution'.
Edward Falkener in his Games Ancient and Oriental (1892) says that Roget issued a card bearing a semi-magic tour of squares and diamonds type, overprinted with the pattern of 16 circuits and bearing the inscription “Key to the Knight's Move as a Magic Square”. The tour shown there is given in Tomlinson Amusements in Chess (1845) but without mention of Roget as its source. We only have Falkener's word that such a card existed; I have not seen the story of the card in any earlier source, and in view of other questionable statements in his book, I am reluctant to accept it as a reliable authority. The tour is both squares and diamonds and Rogetian (see the last section below). The rank sums are far from magic however.
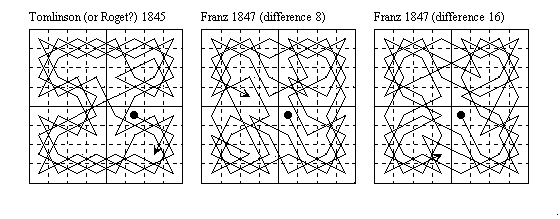
Franz solved the same problem as F.P.H. with closed tours of squares and diamonds type. According to Murray “Franz said that his tours were the nearest approach he had been able to make to a tour with equal-summed rows and columns, and he expressed doubts whether it was possible to do better. It was not then known in Germany that Beverley had already constructed a tour with both rows and columns equal-summed.” His tour with difference 8 is semi-magic, the rows adding to 260±4. His tour with difference 16 adds to 260±2 in rows and columns.
In an article in the Philosophical Magazine in 1840, Peter Mark Roget, who was Secretary of the Royal Society but had not yet published his famous Thesaurus, considered the problem of constructing tours with specified first and last squares (which he believed to be a new condition but which was proposed by Collini in 1773). He claims: “A great many years ago I contrived a method by which the problem in this new and extended form may be resolved with the greatest ease.”

His method divides the board into “four separate systems, of 16 squares each” which he letters L, E, A, P, as in the diagram (actually he used lower case for the vowels). The 168 undirected knight moves on the board are then either of the types LL, EE, AA, PP — these moves form four 4×4 nets, each of 24 moves (we show the LL and AA cases, the PP and EE cases are similar, merely reflected) — or of the types EL, AL, EP, AP, (or their reverse) — these each consist of six strands of three moves (we show the AL and AP cases, the EP and EL cases reflect these, left to right or top to bottom). Roget gave separate diagrams of all eight cases. Moves LP and AE (connecting different consonants or vowels) are not possible.
Roget's method is then to traverse each of the four nets, L, E, A, P, separately, as far as possible, so that the tour is in four parts, except when the start and finish points are in the same net or when the ends of the tour are L and P, or A and E, when it is necessary to traverse one of the nets in two parts.

Roget gave three example tours, as shown. Roget's method satisfactorily solves the problem of presenting a tour as a conjuring trick, since, with practice, a tour can readily be drawn between any two cells of opposite colour quite quickly, even blindfold! Later accounts of the method, with minor refinements, are given by W.H.Browne (1870) and R.C.Read (1959).
It is evident from the above account that Roget's method is quite distinct from the method of Squares and Diamonds. Some tours of squares and diamonds type are Rogetian, but there are many that are not, and moreover most tours constructed by Roget's method are not of squares and diamonds type. Nevertheless, the conflation of Roget's method with the squares and diamonds method is another story which has become almost ineradicable from the literature. This misunderstanding of Roget was pointed out by 'E.H.' in the Glasgow Weekly Herald 1873, where he criticises an account given in the Westminster Papers (which he satirises as the Wastebasket Papers). The confusion may have originated in Charles Tomlinson's treatment of the subject in Amusements in Chess 1845.
H.J.R.Murray (British Chess Magazine 1949) introduced the useful terminology of straights for moves of types LL, EE, AA, PP and slants for moves of types EL, AL, EP, AP. This terminology is applicable to the description of any 8×8 tours, Rogetian or not, and can be extended to other even-sided boards. In this terminology, Roget's method is to construct a tour between the given squares using the minimum number of slants. In retrospect it can be seen that since Collini's circuits are composed entirely of straights, his twelve examples in which minimal deletions are employed to connect the circuits are tours of this Rogetian type.