For asymmetric tours on boards of up to 15 cells see the pages on enumeration of tours on the Smallest Knight-Tourable Boards, Part 1 Open and Part 2 Closed.
The reasons for studying asymmetric tours are various. In enumerating all the tours possible on a given board, some are usually asymmetric, and generally constitute the majority (however there are exceptions, e.g. the 5×6 board). Also of interest are the problems of what board shapes admit unique tours of given types. It is a paradox that some symmetric boards do not admit symmetric tours. Of course if the board is asymmetric then so must the tour be. A series of tours of these paradoxical types (symmetric boards with only asymmetric tours) were given by various contributors to the chess magazine L'Echiquier in the 1920s and 30s.
Included is a selection of Indian 'pictorial' examples some of which use irregularly shaped boards. In Harikrishna (1871), as reproduced by Iyer (1982) these tours are presented on unchequered chessboards, the tour being given by numbered squares within a line drawing depicting the pictorial subject. In his review of Naidu (1922), and in regard to these pictorial tours, Murray (1930) expressed the view that "These appear to me to be of no importance or interest". However, I find them a welcome antidote to too much of a diet of mathematically perfect symmetry. The Indian tours include a study of tours on boards of 32 cells that retain the biaxal symmetry of the 4×8 by cutting out some squares and affixing them in new positions so that the revised board admits a closed tour, which the rectangular board does not.
A more positive term that I have proposed is that an asymmetric tour could be called a "unary tour" since it is one that is all of one piece and cannot be split up into two or more congruent components, as a binary, quaternary or octonary tour can. An asymmetric tour is so-called even if it only has a slight deviation from exact symmetry. Some of the larger tours in fact are constructed to look symmetric, and the asymmetric moves are hidden away in a corner or on opposite edges.
16 cells. The impossibility of a knight tour on the 4×4 board was known in mediaeval times, although no proof was published. Murray (1930) reports: "A Dresden manuscript of the end of the fourteenth century gives a half-tour without solution and sets as a wager game a tour over a board of 4×4 squares" and: "The sixteenth century Persian manuscript on chess in the library of the Royal Asiatic Society makes some remarks on the tour, and promises to give tours on the whole board and on boards of 4×8 and 4×4 squares, which are lost owing to the fragmentary condition of the manuscript. The author boasts a little, for the tour on the 4×4 board is an impossibility."
In his History (1913 p.171, 177, 335 footnote) Murray gives reasons to believe that the author of the work reproduced in this ms may have been Ala'addin Tabrizi (also known as Ali ash Shatranji, i.e. Ali the chessplayer) who is known to have written a work on chess. He was the leading player at the court of Timur (also known as Tamerlane, 1336-1405). A translation of this ms by Forbes (1860) is subtly different, referring to "one quarter of the board" rather than to a 4×4 board. The wording is critical, since a closed tour is possible on one particular non-rectangular quarter-board. There is also a solution on a disconnected quarterboard.

I call the quarter-board tour puzzle 'Aladdin's Conundrum'. However, there is no firm evidence that these quarter-board tours were known before being listed in Paul de Hijo's 1882 catalogue of all the possible 16-move knight circuits in quaternary symmetry. He makes special note of them but does not give a diagram. (I have since found the disconnected case earlier as ¶118 in Le Siècle 16 Mar 1877.) They were rediscovered by H. E. Dudeney (1917) who made them the basis of several puzzles.
Another unique 16 cell tour on a shaped board solves a problem in my booklet on Figured Tours (1997): find a 16-cell knight's tour, on a connected board, showing the first four square numbers 1, 4, 9, 16, forming a square. This can be regarded as a 4×4 board with three corner cells moved.
The 4×4 board admits no complete tour, open or closed. However, moving two corner cells will produce two centro-symmetric boards with equal numbers of dark and light cells when chequered. One of these has four rotary closed tours (see rotary section). The other has no closed or symmetric tours but has 16 asymmetric open tours, all shown here.

17 cells. The problem of a maximum length knight path on the 3×6 board is solved by open tours, omitting one cell.
Andrew Usher [2022] finds eight asymmetric open tours on two board shapes. [Previously we erroneously showed 16-cell tours.]
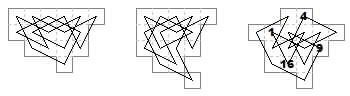
20 cells. The following board, formed from 4×5 with two corners moved has a unique symmetric Bergholtian tour (see rotary section) and 16 asymmetric closed tours (Jelliss 2009). One such asymmetric tour was shown by Godron and Vatriquant in L'Echiquier January 1929 (the last one shown here).
21 cells. Here is an axially symmetric board formed by removing four cells from the 5×5 board. It has no axially symmetric tours, but 51 asymmetric tours.
continued
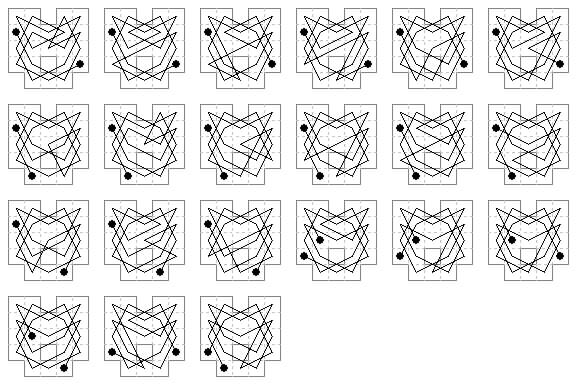
24 cells. Here is a shaped board with two asymmetric closed tours and an asymmetric open tour by Godron and Vatriquant from L'Echiquier Jun 1928 and Jan 1929. A symmetric open tour is impossible since the centre point is not centre of a cell or mid-point of an edge, but this board also has a symmetric closed tour (see rotary section).
We show two other symmetric boards with asymmetric tours (from L'Echiquier Jul and Nov 1928). See the Rotary section for an enumeration of 56 symmetric tours on the first board. Three symmetric closed tours are possible on the other board, all Bergholtian.
On the 24-cell 'top-hat' shaped board a closed tour is impossible, but there is a unique closed pseudotour of two 12-move circuits which can be linked in two ways to form open tours (given by Tolmatchoff and Vatriquant in L'Echiquier July 1929).
Andrew Usher [email 3 December 2022] finds 15 closed tours on the 5×5 board with a corner missing.

28 cells. This amusing 'leotard' board has rotary symmetry but only has the one asymmetric closed tour. There are however two symmetric pseudotours of two types: one in which both components are symmetric, and one in which the two components are the same and are symmetrically related. (There are also 5 symmetric open tours. See rotary tours page).
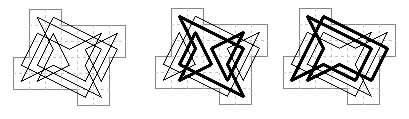
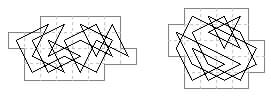
We show two open tours of a 28-cell asymmetric board (formed as 3×4 + 4×4) by Vatriquant and Godron (L'Echiquier Oct 1927). Closed tours are also possible on this board, two examples shown.
An asymmetric board with two closed and two open tours. The other tour on a related symmetric board is from Naidu 1922, representing a peacock.
32 cells. Tours on the 6×6 with four cells cut away. The first is by Euler (1759) second and third by Naidu (1922, #S26). The fouth is an open approximate axial tour by Ambikadatta Vyasa Chaturanga Chaturi (1884), rotated here so the axis is vertical. Naidu (1922) also gives a symmetric closed tour on the 6×6 board with four holes (see rotary section).

On this 'letterbox' board (5×7 with 1×3 hole) the border moves form two circuits, of 8 and 24 moves. Two open tours can be formed as shown by simple linking.
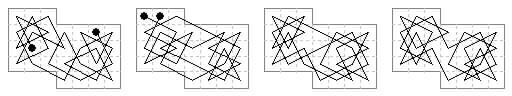
This 5×6 board with two attached cells, is another example that has symmetric open tours and pseudotours (shown here) but on which a closed tour must be asymmetric. The tour shown is by Tolmatchoff (L'Echiquier March 1929).
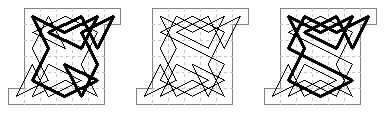
The following tours form part of a systematic study of boards derived from the 4×8 rectangle with some edge cells cut out and reattached elsewhere.

This is a natural subject to study since closed tours on the 4×8 are impossible. The first four boards abovee are biaxially symmetric and the asymmetric tours are #21, #30, #45 and #64 from Naidu (1922). The next three boards only have one axis of symmetry, and the tours are #20, #S51, #S22 from Naidu (the third having holes). The last example, another from Naidu, is biaxial with holes. The diagrams have been rotated 90 degrees to save space.
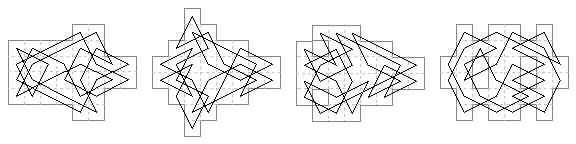
Two more tours from Naidu. The first, like those above, is derived from the 4×8 rectangle with corner cells cut out and reattached to the sides, to make a board with biaxial symmetry, but with an open tour. The second (#S13) is a nearly symmetric tour of a board with diagonal axes of symmetry.
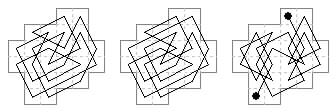
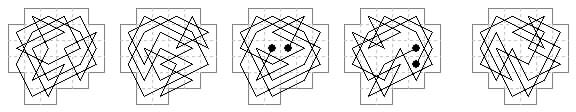
The celtic cross board (above right) with octonary symmetry can be covered by a pseudotour of four 8-move circuits, but has no closed tours, and only two geometrically distinct open tours, differing only by which sides of the dashed lozenge are taken (Jelliss 2003).
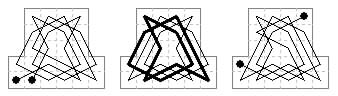
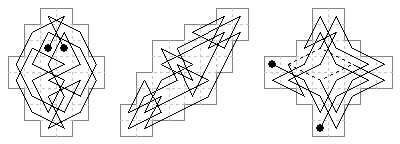
A 32-cell board that makes an interesting case for study is the problem of a tour on the cross-shaped 33-cell solitaire board, where one cell has to be omitted. On the solitaire board of 33 cells there are 17 white and 16 black (assuming the corners are coloured white). A closed tour is not possible because of the odd number of cells. An open tour if possible must start and end on the majority colour white cells, but moves through the black cells a4, d1, d7, g4 form an 8-move short circuit (dotted moves in A), so no open tour is possible.
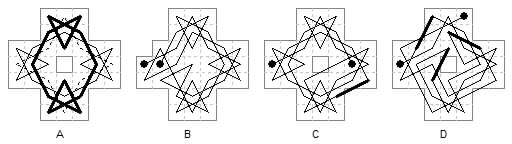
A tour omitting one cell must omit a white cell and must include 7 moves of the 8-move circuit. Because of the octonary symmetry of the circuit we can take a4-c5 as the first move without loss of generality. Under the restriction that moves must be within the area of the cross, the end cell must be on one of b4, c1, c7, e1, e7, f4, and the unused cell at one of a3, a5, d2, d6, g3, g5, since otherwise one or both of the 12-move short circuits shown (in A) are forced. A shaped board solution with start, finish and omitted cells adjacent is shown in (B).
If the unused cell or the end cell is at the centre then the tour must go outside the border at least once, as in (C). The tour in (D) is piece-wise symmetric: when numbered the diametrally opposite cells differ by the constant value 4. [These notes were inspired by a puzzle proposed by Angus Lavery in Games & Puzzles No.2, May 1994, page 34.]
36 cells. The Rajah of Mysore's #45 from Harikrishna (1871) uses this octonary board. Despite appearances the tour is asymmetric.

38 cells. Tour #57 of the Rajah of Mysore in Harikrishna (1871) representing a prancing horse also occurs, reflected, as #33 in Naidu (and #61 is similar).

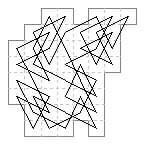
40 cells. More typical Indian tours on irregular boards. The first is #32 in Naidu and represents a yak. The second is #54 in Harikrishna (1871) and #S31 in Naidu (1922) and represents an 'installed Shiva-linga'. The other is #55 in Harikrishna and represents a bull. These two have a single hole.

42 cells. Three more asymmetric pictorial tours. Naidu #15 and #25 represent camel and tiger, and #60 in Harikrisha (1871) representing a vase.
44 cells. First an open tour by Haldeman (1864) with approximate axial symmetry on a cross-shaped board. Second an open asymmetric tour of 8×8 board with five 2×2 voids by Ambikadatta Vyasa Chaturanga Chaturi (1884). Third an asymmetric tour of a symmetric board that cannot be toured symmetrically, by S. Vatriquant L'Echiquier October 1928.

The next tour is the solution of an ingenious puzzle titled 'A Personally Conducted Tour' in Pittsburgh Gazette Times ('Some Puzzles' XVI, 19 Aug 1917) composed by T. R. Dawson and C. D. Locock. On the 8×8 board begin with a Black Knight g8, White King e1. "The knight moves only to check, and may not play into any of the 20 squares cut off round the edges (where it cannot be forced to check). White giving Black no choice of check, makes the knight tour the other 44 squares.">
To force this unusual knight's tour the King has to make a much longer journey: 5 c6, 8 f8, 10 h7, 13 f6, 15 e8, 17 g7, 18 f7, 21 c5, 23 a6, 24 a5, 27 a2, 30 c3, 33 c6, 38 h6, 39 h5, 42 h2, 43 f3, 47 e1, 49 g2, 52 e3, 55 b1, 58 c3, 61 a6, 63 b8, 66 c6, 68 a5, 70 b7, 73 d6, 76 g8, 79 f6, 82 c6, 87 c1, 88 d1, 91 g1, 94 f3, 98 g7, 100 h5, 103 e3, 106 b1, 107 b2, 108 c2, 111 a5, 114 a8, 115 a7, and with 122 King g6, Knight e7+ the tour is made reentrant.
46 cells. Diagram. Naidu (1922) #43 depicting a camel.
48 cells. Closed tour of asymmetric board by Naidu (1922) #46 representing an elephant, and two open asymmetric tours from Indian sources. The first is from Lala Raja Babu Sahib Mo'allim ul Shatranj 1901. The second, on a hatch-shaped board, appears in Ambikadatta Vyasa Chaturanga Chaturi 1884. It joins together four copies of Euler's biaxial 12-cell tour. These tours were collected by H. J. R. Murray (1930).
The first L-shaped tour here is from Eggleton and Eid (1984), formed by simple linking of four edge-hugging circuits; their linkage polygon is noncrossing. My solution alongside keeps within the boundary but the linkage includes a crossover. The third is a squares and diamonds solution (both Jelliss 2016).
50 and 52 cells #8(S) and #10 from Naidu (1922) representing peacock and elephant.

52 cells. Closed tours by H. C. von Warnsdorf (1823) on 7×8 – 4 and 8×8 – 12 boards. Open tour on the same board from Lala Raja Babu Sahib Mo'allim ul Shatranj 1901 (rotated) with approximate axial symmetry. (Closed tours with rotative symmetry are also possible on this board. See rotary section.)
56 cells. Rajah of Mysore #64 board (8×8 – 8).
60 cells. Figure 85 in Wenzelides Schachzeitung (1850), joins four 15 cell tours of the 4×4.
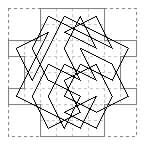
64 cells. This board is formed by combining two identical 32-cell biaxially symmetric boards, but the boards combine asymmetrically, and the tour (Kraitchik 1926 §16) is of course asymmetric. Curiously the board can be dissected into three parts to form an orthodox 8×8 board (Sam Loyd?).

72 cells. A board with octonary symmetry, said to represent a lake with a platform in the centre. Showing an asymmetric tour, #15 by the Rajah of Mysore in Harikrishna 1871. Rotary symmetry is also possible. This is a board within an even frame, 12×12, on which Birotary symmetry is impossible, since 72 = 4×18 and 18 is even.
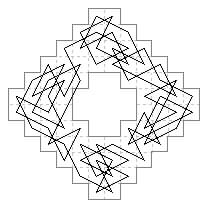
74 cells. This example (#25 from the Rajah of Mysore in Harikrishna 1871) was designed to represent a tree, but nowadays invokes the more ominous mushroom cloud of a nuclear explosion. A similar tour appears as #35 in Naidu (1922). Harikrishna's #28 representing a prancing horse, not shown here, also uses 74 cells, but on an irregular board with two single-cell holes.
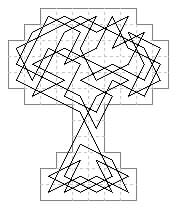
80 cells. These two open tour diagrams represent successive attempts at improved symmetry on the Greek Cross board of five 4×4 components. The first by H. E. Dudeney in Queen 12 Nov 1910: "We select ... one of the more elegant ways of solving this puzzle. Perfect symmetry is not possible, but our path approaches it."It may be noted that the central 4×4 less two cells is based on a closed path with Sulian symmetry, one of two possible. [Cited by Bergholt 1916 who responded with a discussion on symmetry, and more symmetrical examples, open and closed.] This led to the much more symmetrical solution by E. Bergholt in Queen 22 Jan 1916. The linkage k5-l7-j6-l5 of inserted and deleted moves converts it into a pseudotour with biaxial symmetry.

86 cells. This tour by the Rajah of M|ysore #20 from Harikrishna (1871) is said to represent an aerial chariot. The board has axial symmetry but the tour is asymmetric.
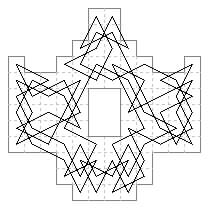
92 cells. Open asymmetric tour on a board 10×10 with 8 cells removed collected by Murray from Zurcher Illustrierte 20 Mar 1931. Has both rotative and diagonal reflective elements.
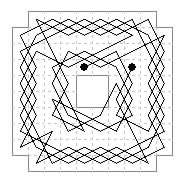
100 cells. Asymmetric closed tour, #23 from Harikrishna 1871, said to represent intertwined serpents. The board is 12×12 minus 44 cells, nearly biaxial. Asymmetric open tour from Zurcher Illustrierte 26 Jun 1931 on a board 10×12 = 120 minus 20 cells. Also nearly biaxial.

On a 10×10 board with the four corner cells moved to the middle of two sides, D. Fiomare in L'Echiquier Nov 1929 gave a simple asymmetric open tour, connecting tours of the four quarters.
108 cells. This 'hash' diagram is an asymmetric tour #17 from Harikrishna 1871, the nine areas removed are described as ponds. (#21 is also on 108 cells but uses an irregular board with a single cell hole, it is said to represent 'an installed Shiva-linga' not reproduced here). Birotary (oblique quaternary) symmetry is possible on this board.
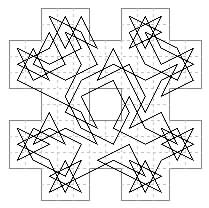
112 cells. Asymmetric tour (144 – 32), #12 from Harikrishna 1871. Oblique binary symmetry is possible on the same board. It does not admit quaternary symmetry.
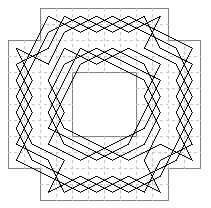
120 cells. Rajah of Mysore: Tour #19 is a 'two-horse movement' each tour covering 60 cells. When numbered the pairs of same numbers occur in adjacent cells, forming a division of the board into dominoes; one pair of dominoes being vertical (30 at h67 and 32 at e67) the others all horizontal. This can be converted into a tour by the linkage: h9-f8-e6-g5-h7-g9.
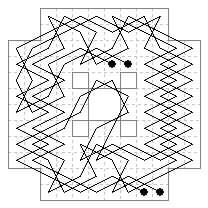
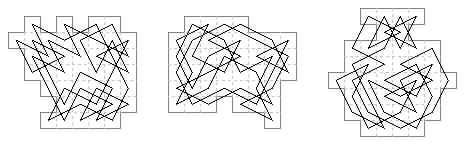
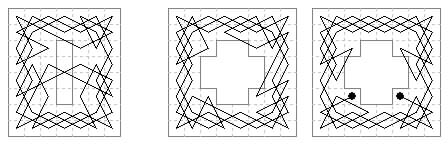
128 cells. The next four open tours exhibit approximate direct quaternary symmetry. The first is from Essener Anzeiger 12 Feb 1933, the others are from the H. Staeker manuscript (1849).

Two others from the Staeker ms:

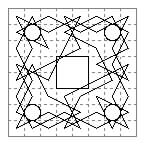
These closed tours from the Staeker ms are very nearly symmetric, appearing to show direct quaternary symmetry, but this of course is impossible on a board within an even by even frame and with an even number of cells in each quarter, and on close examination small flaws in the symmetry can be found. These flaws can be rectified but the result will be a pseudotour. This board is of a type used for four-handed chess.
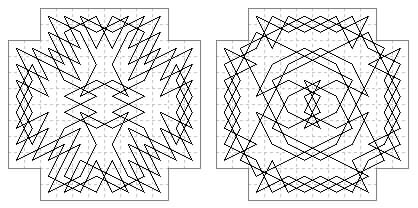
This tour is from G Mann jr (1859). At the end of his collection of chessboard tours he gives this near-axial tour on a four-handed chess board. He also shows a tour on a three-handed board which is similar but with the two ranks at the bottom omitted.
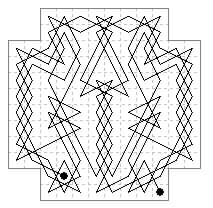
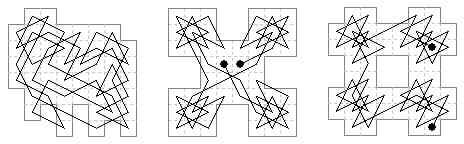
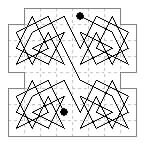
160 cells. The following diagrams are of asymmetric tours on the board for four-handed chess that was popular in the late ninreteenth century. The first two were decoded from hand-written copies of cryptotours taken from Illustrierte Zeitung for the period 1852-63:
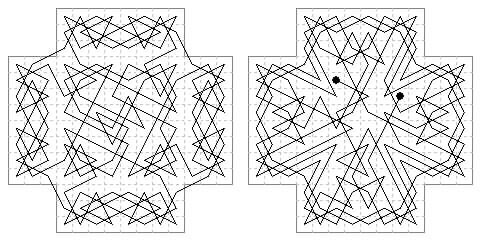
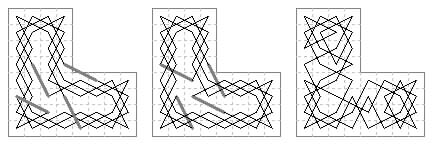
The next is 'The Knight's Tour on the Board Used in Chess for Four Players' Frontispiece to Vol 3 of Westminster Papers 1871.
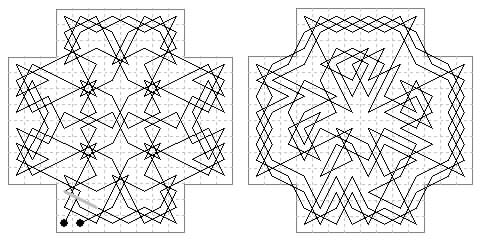
The above frontispiece tour is described as 'a figure of the greatest beauty and regularity' and the author as Victor Gorgias 'of Oëdenberg'. It shows the maximum amount of direct quaternary symmetry possible in an open tour (and also a high degree of octonary symmetry). If the move marked in grey is deleted and the two loose ends of the move are connected to the ends of the tour the result is a pseudotour.
The closed partially symmetric tour is by A. H. Frost (1877). He also gives a tour by the compartmetal method, joining up ten tours 3×4 and two 4×5 on this board.